Mathematics - 2017-11-17 (page 8 of 8)

11:08 PM

Quick question for algebraic geometers: when talking about "a local ring whose completion is normal" (en.wikipedia.org/wiki/Analytically_normal_ring) is it always assumed that the completion is with respect to the maximal ideal?

Ted Shifrin

@Damin Demonark, yes, you're completely correct. However, the converse fails, as you know, too.

@rschwieb: I'm not an algebraic sort of algebraic geometer, but it seems to me that's the only thing it can mean, yes. :)

rschwieb

It seems like the wiki articles on "completions" never state this explicitly, but it seems like the most likely meaning.

@TedShifrin thanks for pitching in your two cents...

Ted Shifrin

Eisenbud and such texts are a lot more reliable than Wiki, but I no longer have mine to confirm for you.

ALannister

I love your avatar @rschwieb is it a guy panicking?

Ted Shifrin

@rschwieb: Lang says "By a complete local ring, one always means a local ring which is complete in the $\mathfrak m$-adic topology." ($\mathfrak m$ being its unique maximal ideal)

Meow Mix

11:14 PM

hi

Ted Shifrin

Heya Meow.

Manolis Lyviakis

why checking if loops are equivalent if the shrink to the same point ?

the7*

they*

Ted Shifrin

That's too vague a question.

BTW, within a few minutes, you can edit your post and correct typos.

Victor

Hi,were anyone here taking probability course can help me on this one? math.stackexchange.com/questions/2525418/…

Ted Shifrin

@Victor: You should be able to do that yourself. You need to know the definition of expectation or expected value.

Manolis Lyviakis

11:20 PM

Say there are 2 loops of the same point. I wanna check if they are equivalent.Our professor used as an argument they shrink to that point so all the possible classes is just one

without rigor

Alessandro Codenotti

Hi chat

Manolis Lyviakis

to be equivallent must there be a homotopy between them

Meow Mix

ted this problem is stumping me

Manolis Lyviakis

so a deformation to one each other

Ted Shifrin

What do you mean by equivalent, @Manolis? Homotopic?

Manolis Lyviakis

11:22 PM

yeap

Ted Shifrin

If the space is simply connected (which is what allows you to shrink any curve to a point), then every closed curve is homotopic to a point, yes.

@Meow: That's a bit vague.

hi, @Alessandro

@Manolis: What is your space?

Manolis Lyviakis

what does shrinking mean

homotopy is deformation of a path to another

why shrink them?

Ted Shifrin

It's intuitive ... shrinking usually means you use the straight-line homotopy to your point.

Manolis Lyviakis

ohh so there is a homotopy from a path to a point?

MatheinBoulomenos

a point is a constant path

Manolis Lyviakis

11:24 PM

ok thats what i wanted

Meow Mix

i just odnt know where to start

all my solutions prove futile

user104729

@ManolisLyviakis Haven't you done $\pi_1(\Bbb S^1)$, $\pi_1(\Bbb S^2)$ for intuition

Manolis Lyviakis

not yet

Meow Mix

i can write out the coefficients of the sum out of the coefficients of $f$ but that proves futile

Manolis Lyviakis

only basic definitions

of homoty and fundamental groups

next lecture is calculating some funtamental groups

Im an undergraduate i think for a basic undergraduate topology class he has gone abit far

hell go as far to jordans theorem proof

user104729

11:28 PM

@ManolisLyviakis Well, you can think of a rubber band on a sphere, and since there are no obstructions, it will 'contract to a point'. But if the rubber band is stuck around a circle (think of a string tied around a pole, that has infinite length if you wish) and the rubber band can not be taken away.

Ted Shifrin

@Meow: Have you told us your question?

Meow Mix

it was the second one you said in your email

Ted Shifrin

Ohhhh ... I was supposed to know that?!!

Meow Mix

if polynomial $f \ge 0$ for all $x$ then $f + f'' + f'' + f''' + \dots \geq 0$

Ted Shifrin

Yes, I remember.

So what do you know about the degree of $f$?

Manolis Lyviakis

11:30 PM

@user104729 you are saying that any loop on sphere that doesnt go around the sphere can be taken away but if it does a whole round it cant?

user104729

@ManolisLyviakis Just think of a rubber band on a sphere in the real world. I am just saying, that you can shrink the rubber band to a point.

Ted Shifrin

@Manolis: It's going to turn out that any closed loop on a sphere is homotopic to a constant loop (point). But it's not totally obvious how to prove it.

user104729

The rubber band is a loop on the surface on the sphere.

Ted Shifrin

@Meow: Are you answering me?

Meow Mix

sorry i was doing something

ok so

the degree of $f$ is even

and it has a positive leading coefficient

Ted Shifrin

11:33 PM

Let $g=f+f'+f''+\dots$ ... What can you say about $g$?

Meow Mix

it also has an even degree?

Ted Shifrin

OK. Now what have you proved in previous chapters about odd- and even-degree polynomials?

(I already gave you that hint a few days ago, but you probably ignored me, as usual.)

user104729

@TedShifrin Savage :P.

Ted Shifrin

shrug

@user104729: You see now why I'm being "savage." Did he pay attention? Is he looking? I have no clue. @Meow

user104729

@TedShifrin Haha, I don't think it was rude. I just thought it was amusing. I feel like Meow Mix is thinking about it, and pretending not to, so his delay doesn't make you think he is stupid.

I've been there for sure.

Ted Shifrin

11:40 PM

Usually, it's good to get a one-word response like "Aha" or "Thinking" :P

user104729

I agree :).

@BalarkaSen Hahaha, this comment is gold.

Ted Shifrin

Balarka is capable of being rude/blunt, too :P

Meow Mix

sorry i was on a skype call

okay so what did we prove

Semiclassical

@ted a small but cute observation re: my surface from earlier (the unshifted version)

Mike Miller

he learned from me, i think

rip

Ted Shifrin

11:48 PM

LOL, Mike.

@Meow: That's for you to figure out.

Leaky Nun

poll: what's your favourite visualization of $\Bbb S^3$?
1. as $\widehat{\Bbb R^3}$
2. as $\Bbb D^3$ with the boundary identified to one point
3. as $\Bbb D^3 \cup \Bbb D^3$ with the boundaries glued to each other
4. as two solid tori with boundaries glued to each other in the wrong way
5. others

Ted Shifrin

Remember I told you that I went back and taught Chapters 7 and 8 before doing chapter 11. :) @Meow

Semiclassical

As noted earlier, it contains (1,1,1) as the vertex of what is locally a cone. Nothing new there.

Meow Mix

alright im just gonna leave this cal for now

Mike Miller

$S^3$ looks the same as $S^2$

It's just a bit bigger

Leaky Nun

11:50 PM

yeah right

MatheinBoulomenos

Definitely 4

user104729

@MeowMix wut?

Leaky Nun

I like 4 too, but 4 and 1 are kind of connected

Ted Shifrin

All of the above, Leaky, depending on what's going on.

user104729

@MeowMix Isn't it freshest on your mind?

Meow Mix

11:50 PM

huh?

Mike Miller

@user104729 Not calculation, but rather call as in Skype call

Ted Shifrin

@Leaky: I often prefer the unit sphere in $\Bbb C^2$.

user104729

Oh hahahaha

Right @MikeMiller.

Leaky Nun

like for 1 I would further decompose it into a base circle, continuumly many tori, and then a pole in the middle

Mike Miller

I went through the same thought process ;)

Leaky Nun

11:51 PM

@TedShifrin your mind must be able to visualize 4D space :D

Ted Shifrin

@Leaky: I already told you that complex geometers think in real pictures, understanding what they're doing.

Leaky Nun

actually what I just said for 1 is essentially the Hopf map video

MatheinBoulomenos

unit quaternions, as this gives a group structure

Ted Shifrin

Yup, Mathei.

I won't even argue this time :)

Semiclassical

But at the level of the equation it’s symmetric under the reflection (x,y,z) -> (-x,-y,z) and is symmetric under any permutation of the coordinates

Ted Shifrin

11:52 PM

Although I usually would say $SU(2)$. :P

Mike Miller

One should try to give optimally irritating definitions

$\Omega \Bbb{HP}^\infty$?

2

Leaky Nun

HP @_@

Ted Shifrin

@Semiclassic, I agree on permutation, but I don't agree on the two minuses.

Semiclassical

Which I think leads to it having the same symmetry group as a regular tetrahedron

Mike Miller

harry potter and the group structure on a loop space

4

Ted Shifrin

11:53 PM

Up to rotation of coordinates, we already said it was $z^2 = 2(x^2+y^2)$. :P

Leaky Nun

if $\Bbb S^3 / \Bbb S^1 \cong \Bbb {CP}$, then does $\Bbb S^7 / \Bbb S^3 \cong \Bbb {HP}$?

Ted Shifrin

Yes, Leaky.

Mike Miller

stick some 1s in the exponents but yues

Ted Shifrin

You're missing $^1$s.

Leaky Nun

is it true that $x^1 = x$? :P

Semiclassical

11:54 PM

Yes, after shifting coordinates to be centered st (1.1,1)

Ted Shifrin

@Semiclassic: A cone has a lot more symmetries than a tetrahedron. There's a whole continuous group.

There's an obvious $O(2)$.

Leaky Nun

what is the symmetry of the whole space? SO(3)?

Ted Shifrin

$E(3)$?

Leaky Nun

Euclidean group

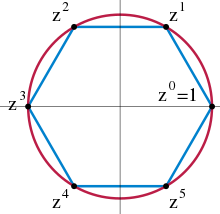
In mathematics, the Euclidean group E(n), also known as ISO(n) or similar, is the symmetry group of n-dimensional Euclidean space. Its elements are the isometries associated with the Euclidean distance, and are called Euclidean isometries, Euclidean transformations or Rigid transformations. Euclidean isometries are classified into direct isometries and indirect isometries, an indirect isometry being an isometry that transforms any object into its mirror image. The direct Euclidean isometries form a group, the special Euclidean group, whose elements are called Euclidean motions, displacements or...

Semiclassical

But the original unshifted (and unapproximatef) version was x^2+z^2+z^2=1+2xyz

Leaky Nun

11:55 PM

O(n) = E(n)/T(n)

fair enough

Ted Shifrin

@Semiclassic: I'm not following you.

Are you referring to that version?

I see.

So with the cubic in there you're saying it has those symmetries. I missed that.

But you're talking about symmetries relative to the origin, not relative to the cone point.