The h Bar

8:00 PM

Note it literally admits there is 'one magic step' which can't be avoided in transitioning from classical to quantum which is an averaging which is simply unjustifiable classically and amounts to the normal QM theory

Semiclassical

Now, suppose you had a trajectory for such a system

enumaris

@vzn GUT only seeks to unify the strong, weak, and electromagnetic forces

it doesn't deal with gravity

bolbteppa

@vzn the problem is those people you call elitist's who you think are afraid of new theories have looked into all of this stuff for years properly and not just read headlines without understanding the math

vzn

@enumaris btw wondering if you have any asian bkg or familiarity? taoism? (zen) buddhism? en.wikipedia.org/wiki/Shoshin

Semiclassical

To the extent that $m$ is very small, can you measure two different trajectories with the same initial position and different initial velocities?

enumaris

8:01 PM

I studied buddhism and hinduism in college

vzn

@bolbteppa lol havent used the word elitist in at least 5 min :P

bolbteppa

@vzn blue's point was for you to justify yourself, not link people to obscure references

enumaris

@vzn I think your repeated mention of the hbar chat and the users here and all that interplay makes it hard to read your blog as a physics blog

bolbteppa

e.g. where in Baggott is it 'derived' and what point are you trying to make in me reading this 'derivation'

enumaris

also, you need to define what you're talking about because there's a bunch of stuff on there that I don't know what you mean

Semiclassical

8:03 PM

the problem is that, in as much as the trajectory is defined by dx/dt=1/b dU/dx, the left-hand side at t=0 is determined by the value of x

vzn

@bolbteppa blue faded away on being further challenged presumably with good reason. aka irredeemably superficial™ :P

Sid

@BalarkaSen indeed. Loved every second of the Second ahalf

Semiclassical

@vzn oh ffs. people have lives

Balarka Sen

@Sid Very much

It was a good game

vzn

@Semiclassical yeah am thinking strongly lately should get one too away from this chat :P

Semiclassical

8:04 PM

not everyone has the time or the patience to sit around here debating with you

enumaris

@vzn also you mentioned Balarka left the hbar in protest, and yet here he is ^

bolbteppa

@Semiclassical can you summarize the point of that?

Semiclassical

@bolbteppa my point is that, in as much as the trajectory is defined by that equation of motion, the initial velocity and the initial position are not independent quantities

vzn

@enumaris lol/ sigh think theres tons of physics in it, let me know when you find any :P

Semiclassical

the moment you measure the initial position of a particle subject to such strong damping, you can infer the velocity

enumaris

8:05 PM

yeah but if you want to get your point across, it's generally not good to obscure it in a field of other stuff

It's why physics papers don't have long sections about all the stuff that's happening in the author's physics department

Semiclassical

As an example, suppose that it's a harmonic potential. Then one has $m\ddot{x}=-b\dot{x}+kx$, so in the limit of strong damping one has $\dot{x}=kx/b$

vzn

@enumaris sometimes its pretty hard getting a point across even while attempting to get a point across. havent written an arxiv paper recently on the content. will let you know when it happens. maybe you want to wait for that.

enumaris

unfortunately, I read the entire blog, but I was unable to ascertain what exactly the "fluid paradigm" really is

Semiclassical

so if you measure the initial position as $x_0$, then the initial velocity is constrained to be $v_0=kx_0/b$

vzn

@enumaris lol too bad. guess its now an est pattern with blue + semiclassical + BS et al :P

enumaris

8:07 PM

there do seem to be nuggets of interesting points scattered across though

but I also can't see how they tie in to each other

Semiclassical

Now, of course, this remains a classical system subject to Newton's laws. The trick here is that, if the initial velocity at initial position $x=x_0$ differs from $kx_0/b$, then after a very small time has passed one has $v(t\gtrsim 0)\to kx_0/b$

vzn

@enumaris thx for trying! :)

enumaris

well, I'm only commenting on my understanding of the blog, I have not enough understanding to comment on the content of the blog beyond the parts about how to get the point across more clearly.

Semiclassical

as such, the true initial velocity is hidden by the short time scale in this system

vzn

@enumaris its a new paradigm, it will feel strange/ incomprensible at 1st. have been working on it on the blog alone (intermittently, although more recently) over ½ decade now.

enumaris

8:09 PM

It's not really about strangeness, I don't mind strangeness, but there needs to be some definitions at least...

Semiclassical

@bolbteppa overall, the point is that there are two ways you could understand an equation of motion like dx/dt=dU/dx

Anonymous

@Sid I just watched it for 10 mins but the header from the Belgian side was excellent. Can't recall his name

Semiclassical

one is to insist that there really is an inertial term and therefore the trajectory is described by classical mechanics.

bolbteppa

@Semiclassical this is all clearly an approximation of Newton's laws, we're talking about the very fundamentals

vzn

@enumaris will endeavor to walk you thru it over time "as time/ patience permits™" :)

enumaris

8:11 PM

Like what exactly it means for QM to be a "fluid theory". Is it only that you expect the equations from Fluid Dynamics can be applied analogously to QM's framework to make predictions? Or that you have to e.g. reinvent the entire framework of QM to be more of a classical fluid-type theory?

Semiclassical

the other is to treat dq/dt = dU/dx as the more fundamental equation

Sid

@Blue The 1st goal? I think It was Vertonghen

vzn

@enumaris many questions. many excellent (shoshin) questions. let me start by putting it this way, "answering" your question with another question. what is the defn of the copenhagen interpretation?

Semiclassical

i.e. to say that dq/dt = dU/dx is itself a perfectly good equation of motion and refuse to insist that there's an inertial term

bolbteppa

The initial position and velocity are independent quantities, saying they aren't is like saying a linear second order ode does not depend on two constants of integration

Semiclassical

8:13 PM

yes, insofar as we're talking about a linear second order ODE

bohmian trajectories are not governed by a linear second order ODE

they're governed by a first order one

now, you can insist that the first-order law they obey is really an approximation of some second-order law

enumaris

I would say it's a minimalist way of understanding the equations of QM...but it is indeed hard to give a clear and concise definition.

Semiclassical

that's basically how PWH is supposed to work.

vzn

@enumaris agreed, & (maybe?) it is hard because its originator, bohr, never gave a clear/ concise defn himself o_O

Semiclassical

Bohmian mechanics as such, though, does not treat the first order law that the trajectories obey as being some approximation

it treats the first order law (ye olde guidance equation) as the end of the story as far as what determines the trajectories

Anonymous

@Sid Ah, yes

enumaris

8:16 PM

but 1. the copenhagen interpretation is an interpretation of QM and therefore not a physical theory in and of itself. 2. Just because Bohr never gave a clear and concise definition himself does not mean that a physical theory should not be clearly defined.

vzn

@enumaris now, my "answer" is, the copenhagen interpretation is an overarching interpretation of QM in the same way the fluid paradigm is for this new theory. have another (earlier) blog on that when youre ready.

enumaris

so your fluid paradigm is an interpretation only?

kinda hard to come up with an interpretation before you even have a theory tho isn't it...

After all, QM interpretations popped up only after QM was itself established

vzn

@enumaris its a "guiding principle" with many corroborating elements so far. it starts out as a new interpretation that has to be adopted/ pushed (mainly by advocates/ adherents) to gradually grow into a more complete theory.

DanielSank

Hi, everybody.

I need help.

enumaris

hello

DanielSank

8:18 PM

Suppose I have a harmonic oscillator.

Semiclassical

I'll note that, in the context of Bush-Couder's work on bouncing droplets as quantum analogues, the distinction between "pilot wave hydrodynamics" vs. "Bohmian trajectories" vs. "quantum mechanics" is quite clear

DanielSank

Suppose the classical Hamiltonian is $$H = \frac{\Phi^2}{2L} + \frac{Q^2}{2C} \, .$$

vzn

@enumaris the copenhagen interpretation was grounded/ preceded by earlier bohr philosophy such as the complementarity principle. so maybe the fluid paradigm is more comparable/ analogous to the complementarity principle. etc.

DanielSank

The equations of motion are $$\dot{\Phi} = Q/C \qquad \dot{Q} = -\Phi/L \, .$$

Semiclassical

pilot-wave hydrodynamics in such a system is the 'fundamental' description of what's going on, giving the behavior of the droplets at a fast time scale

enumaris

8:20 PM

ok...

Semiclassical

if you consider a bunch of such droplet motions, though, then you can take ensemble averages of such paths

DanielSank

This can be written as a matrix $$\frac{d}{dt} \left( \begin{array}{c}\Phi \\ Q \end{array} \right) = \left[ \begin{array}{cc} 0 & 1/C \\ -1/L & 0 \end{array} \right] \left( \begin{array}{c} \Phi \\ Q \end{array} \right)$$

enumaris

so what would the "principle of the fluid paradigm" be? "All physical laws can be formally made into analogy with fluid-dynamical laws"?

vzn

@enumaris the complementarity principle is rather nebulous in various ways yet helped guide research in a key/ helpful way.

enumaris

So is Mach's principle

bolbteppa

8:21 PM

@Semiclassical I will try read up on Bohm stuff more over the next while, have a well-defined issue to try sort out now at least, maybe we'll come back to this another time if I can't get rid of this sense myself

Semiclassical

in which case there will be well-defined trajectories at an intermediate time scale subject to short-time fluctuationso

enumaris

nothing wrong with a guiding principle being vague and nebulous I guess, but there's gotta be at least a principle...

vzn

@enumaris it says that the old ether idea was not entirely wrong and that there is a "neo" fluid ether going by various names eg "spacetime fabric, madelung fluid" which all are the same thing and we are on the verge of unifying them. etc. it says many other things but havent put them in a simple list yet.

DanielSank

Interestingly, the eigenvalues of that matrix are $\pm i \omega_0$ where $\omega_0 \equiv 1/\sqrt{LC}$ is the oscillation frequency of the oscillator.

Semiclassical

if you then do long-time statistics, you get the same quantities which everyone agrees about re: QM

e.g. Born's rule holds at all times

vzn

8:23 PM

@enumaris just googled that, kind of obscure. some new emergent theories of gravity are not so different and as mentioned in the blog, do think they align nicely with the fluid paradigm.

DanielSank

This is quite compelling. Here we're saying that a matrix associated with the Hamiltonian equations of motion give the frequencies, and Planck found that $E = \hbar \omega$, so it seems that the idea that the Hamiltonian in quantum mechanics have eigenvalues that give the system's energy levels isn't that weird.

Semiclassical

So within the droplet model one has fast/medium/long-time scale descriptions

DanielSank

Discuss.

enumaris

@vzn so the general gist of the principle is that the analogies between fluid mechanics and other fields is more than just formal, mathematical analogies, but can be taken as expressions of some underlying physical mechanism?

DanielSank

@Qmechanic @ACuriousMind @EmilioPisanty see a few messages up.

vzn

8:23 PM

@enumaris YES etc! ty! :)

enumaris

ok

bolbteppa

One interesting thing regarding all this is I went over how a free photon coordinate space wave function is literally impossible, it can only specify the position to within some region (non-local), but it's momentum space wave function is perfectly fine, and literally the only thing one can measure

Semiclassical

with the long-time scale description being analogous to QM, the medium time scale to Bohmian mechanics, and the fast time scale being different from both

enumaris

as a guiding principle it doesn't seem worse than many of the others out there used to get to real physical theories

Semiclassical

It's this last one which I take to be PWH in the Bush-Couder sense

bolbteppa

8:24 PM

If I was really into Bohm stuff I would see this as a fatal flaw etc

Semiclassical

As a system, I think it's neat

enumaris

but it's quite difficult (massive undertaking) to go from here to a comprehensive physical theory XD

vzn

@enumaris think it is already proven better but it hasnt collectively sunk in anywhere yet except maybe in my own neurons :)

enumaris

well, I wish you luck in your endeavor

Semiclassical

@bolbteppa eh, once we're doing photons we're doing QED and then I dunno wtf one should say

bolbteppa

8:25 PM

The arguments for this nearly ended qed in the 30's, took Bohr like 2 years to answer it

In fact his answer is a 60 page behemoth which saved QED only by technicalities

vzn

@enumaris thx, am not alone, but still need or at least could use your help :)

bolbteppa

qed is almost literally impossible it's so delicate apparently

Semiclassical

and it's here I'll note that I find the Bohmian story to be consistent in the context of non-relativistic QM

enumaris

I gotta say, I was never much of a fluid mech guy myself

Semiclassical

once you start doing stuff which is supposed to be relativistic, then the story I gave is definitely not going to work

enumaris

8:26 PM

my understanding of the Navier-Stokes equation for example is rudimentary at best

vzn

@DanielSank have a posted Physics relating to that analogy, think it is more than an analogy™

enumaris

would take a long time just to read through all the material making those analogies lol

Semiclassical

and I'm basically not sure whether one should even attempt to make Bohmian trajectories work outside the context of non-relativistic QM

enumaris

the answer is yes

one should attempt it

vzn

@enumaris there is an old saying by rumsfield (architect) about iraq war. you go to war with the one you have, not the one you want™ ... he said other zen things about "known knowns" etc :P

enumaris

8:28 PM

even if in the end one simply ends up with an alternative formulation of QFT, it's generally true that new insights are learned from alternative formulations

Semiclassical

ugh rumsfeld

But my insistence is not that Bohmian stuff is "the true theory". it's that the Bohmian interpretation of non-relativistic QM is internally consistent and experimentally equivalent to the standard QM story

that's enough for me to find it interesting.

vzn

← got a close-shave C in sr undergrad fluid dynamics class, one of my 2 hardest in college

Semiclassical

I read that momentarily as 'special relativistic fluid dynamics' and was like "that was an undergrad course?!?"

vzn

@enumaris GUT + TOE researchers are currently wandering in the wilderness and increasingly know/ admit it (actually not a lot has chged since einstein RIP ½ century ago). a guiding principle is a path. and again, there are very many corroborating facets already established.

bolbteppa

But Bohm stuff should be the true theory

If it is going to correctly describe things and make logical sense

Semiclassical

8:33 PM

uh, why? it only has to make sense within its domain

that's like insisting that QM is a bad theory because it doesn't work for describing quantum gravity

vzn

@enumaris highlighted a ref in my blog that derives sch eqn from navier stokes. (there are a few along these lines.) the bridge is apparently madelung fluid

bolbteppa

It took me a long time to make sense of QM so I have patience with Bohm until I make sense of it (if it makes any :p)

Semiclassical

For completeness, I'll note that the suggestion Bush puts forward for the dynamics that 'should' underlie QED (in the same way that the fast-scale droplet motion gives rise to intermediate- and long-time dynamics) is so-called stochastic electrodynamics

with the fast-scale in that story being at the scale of Zitterbewegung

...I find myself rather skeptical of it

bolbteppa

The problem is clearly gravity which does not possess a quantum description yet, and suffers the exact same problem the Fermi decay model suffered r.e. renormalizability but being of different spin enormously changes things

(and gravity does have a quantum description in strings :p so it's already been seen to be possible hah)

I would love to see what Bohr etc said of Bohm, I know he had to go to South America partially over it :p

Semiclassical

I know what Einstein said. He thought Bohm's approach was "too cheap" :P

my point anyways more: You'd never insist that the Schrodinger equation is a one-size-fits-all description of particle physics

heather

8:39 PM

@BalarkaSen, yeah, I kinda found it interesting myself...if i have some free time i might look into it more =) thanks

Semiclassical

it's a useful equation within its domain i.e. non-relativistic QM

bolbteppa

The Schrodinger equation applies to qed and qft

It's not just non-relativistic, which has me even more curious as to why it fails for Bohm

Semiclassical

so you're saying that the wavefunction satisfies an ODE which is first-order in time, second-order in space, regardless of whether you're doing QED or not?

vzn

@Semiclassical again, encourage you to see it (bohmian mechanics) as a bridge somewhat in spirit of Bohrs provisional hydrogen electron "orbits".

Anonymous

@DanielSank That's an interesting observation, but not very unexpected I suppose. We transition from classical phase space to Hilbert space by replacing the commutation relation (poisson bracket) $\{\Phi,Q\}=1$ with $[\Phi,Q]=i\hbar$. Notice, you got the eigenvalues of that as $i\omega_0$ while in the quantum case the eigenvalue would turn out to be $E=i\hbar \omega_0$. That extra $i\hbar$ comes precisely from the commutation relation (as far as I understand)

bolbteppa

8:41 PM

No, the actual form of the Hamiltonian is derived from Galilean symmetry in the non-relativistic case, but the Schrodinger equation is more fundamental than that, doesn't matter what form $H$ takes, which is why we get Klein-Gordon, Dirac, Maxwell etc following it

Semiclassical

hmm

If you mean Schrodinger as an umbrella term that includes K-G, Dirac, etc

then sure

I'm specifically meaning the form it takes in the non-relativistic setting. One would not insist on that in the general case

Anonymous

Quantum LC circuit

An LC circuit can be quantized using the same methods as for the quantum harmonic oscillator. An LC circuit is a variety of resonant circuit, and consists of an inductor, represented by the letter L, and a capacitor, represented by the letter C. When connected together, an electric current can alternate between them at the circuit's resonant frequency: ω = 1 L C {\displaystyle \omega ={\sqrt {1 \over LC}}} ...

Balarka Sen

@heather

Anonymous

@DanielSank Small typo there. I meant $E=h\omega_0$

Anonymous

No iota

bolbteppa

8:45 PM

Another thing is this whole 'no particles only fields' thing, I'm pretty sure that's not true

Semiclassical

@bolbteppa I'll also note (with some distaste) that at least one proponent of Bohmian mechanics argues that it's not even a Galilean spacetime but an Aristotelian one

which...ugh

bolbteppa

Haha, can you imagine Aristotelian qft

Semiclassical

lol

enumaris

@vzn when I have time I'll look into the Madelung equations

right now I'm trying to read Landau and Lifshitz though

bolbteppa

Read section 1 of volume 4 of L&L on amazon

It's like 3-4 pages

Semiclassical

8:47 PM

@bolbteppa here's the source on that: arxiv.org/abs/0812.4941

bolbteppa

amazon.com/Quantum-Electrodynamics-Second-Theoretical-Physics/…

vzn

@Semiclassical lol afaik aristotle, "very early physicist," postulated the ether :P en.wikipedia.org/wiki/Classical_element

Semiclassical

which is one of those papers which makes me leery of considering myself a Bohmian

bolbteppa

He literally says all you can measure is free particles, and the idea of measuring interacting particles in qft is as unreal as paths in QM are

vzn

@enumaris cool thx np everyones busy :)

bolbteppa

8:48 PM

and gives justifications beforehand based on the relativistic HUP

enumaris

mmm

bolbteppa

I think that is simply insane and shocking

Semiclassical

@bolbteppa The closest thing I've seen to a counterpoint re: Bohmian mech and QFT is this one: arxiv.org/abs/1307.1714

vzn

← except for me took 1wk vac this week, am sure everyone else in here is delighted :P

danielunderwood

What even is Aristotelian spacetime?

enumaris

8:52 PM

aristotle didn't know no spacetime

danielunderwood

I found something on google, but it just looks like Galilean at first glance

enumaris

Aristotle thought object's "natural state" is "at rest"

that's certainly not Galilean

Semiclassical

see page 20 of these lecture notes: tcm.phy.cam.ac.uk/~mdt26/PWT/lectures/bohm5.pdf

bolbteppa

Aristotle thought velocity not acceleration was the 'generator' of motion or something

Semiclassical

yeah

enumaris

8:53 PM

In other words, I think Aristotle's "space time" would be one which has a preferred reference frame of the Earth, and objects naturally tend towards 0-velocity in this preferred frame.

heather

@BalarkaSen what's that a screenshot of?

Semiclassical

and tbh that is pretty much true in the Bohmian mechanics story

enumaris

but he wouldn't have had any notions of a reference frame

Semiclassical

that's what I'm on about it being a first-order theory

enumaris

that's a modern take at some of his ideas

Balarka Sen

8:54 PM

@heather Contents of Gromov's text "Partial Differential Relations"

Semiclassical

now, you can find that first-order property distateful

but it's not an inconsistent one

Balarka Sen

I have been looking into those for quite some months now. So you see the reason for my interest in your question :)

heather

@BalarkaSen, ah, okay, let me look it up

enumaris

you will BOW to the power of the FIRST ORDER

Balarka Sen

I don't recommend trying to read that book

heather

8:55 PM

@BalarkaSen oh, really? any particular reason?

bolbteppa

Non-local is still a buzz-word to me beyond a few examples of square roots of differential operators :\

Semiclassical

But ugh. assuming that the universe has a privileged rest frame is...yuck

Balarka Sen

@heather I can explain what partial differential relations and h-principles are if you have time :)

I suspect the excitement will be tautological after the explanation

heather

i think i do, and i would be very interested.

Balarka Sen

A'ight.

Semiclassical

8:56 PM

And insofar as that route seems to be what PWH would insist upon, I find myself reluctant to countenance it

To put it a little differently: In Bohmian mechanics, there's this notion of 'quantum equillbrium'

heather

(geesh, i can tell why you said you didn't recommend trying to read that book)

vzn

@Semiclassical being flexible in semantics, that is essentially exactly the assertion/ construction of Tenev + Horstemeyer and it is not ugly at all, it is basically equivalent to conventional GR.

enumaris

what about the rest frame of the CMB, it's somewhat special in modern cosmology...tho it's not "privileged" in the normal physics sense

Semiclassical

The nice thing is that, if this equilibrium condition is fulfilled at any time, then the unitary evolution of the Schrodinger equation guarantees it's fulfilled at any other time

and that basically guarantees that the Born rule is satisfied for all times

Emilio Pisanty

@DanielSank ugh, that's starting off on the wrong foot

Semiclassical

9:00 PM

Some workers on pilot-wave stuff like the idea that there could be systems in quantum non-equilibrium

Emilio Pisanty

I never could get the hang of the whole "$\Phi$ and $Q$ are canonical conjugates" thing

Semiclassical

in which case you'd see definite departures from standard QM predictions

Balarka Sen

@heather A partial differential equation, remember, is an equation $$\displaystyle \Phi \left (f, \frac{\partial f}{\partial x_1}, \frac{\partial f}{\partial x_2}, \cdots, \frac{\partial f}{\partial x_n}, \frac{\partial^2 f}{\partial x_1^2}, \frac{\partial^2 f}{\partial x_1 \partial x_2}, \cdots, \frac{\partial^r f}{\partial x_{i_1} \partial x_{i_2} \cdots \partial x_{i_r}}\right ) = 0$$ of the various mixed partial derivatives of $f$ of different orders.

possibly with boundary conditions on the multivariable function $f(x_1, \cdots, x_n)$ on whatever domain $\Omega \subset \Bbb R^n$ you want to solve the PDE on.

Semiclassical

I am far more tepid on that, insofar as it'd allow you to have measurable violation of Lorentz invariance

Emilio Pisanty

ah. luckily it's not actually required.

Semiclassical

9:01 PM

which...yuck

Emilio Pisanty

@DanielSank Is that really what you're seeing, though?

bolbteppa

Apparently non-local is sometimes a way of saying Lorentz invariance fails or something

Semiclassical

my own gut feeling (which I can't validate and probably will never be able to) is that the equilibrium hypothesis is fundamental

Emilio Pisanty

or are you seeing the classical analogue of the fact that $[H,a]=\hbar\omega a$?

Semiclassical

i.e. that our inability to see violations of it is a matter of principle, not of circumstance

Emilio Pisanty

9:02 PM

i.e. that $\hat a$ is an eigenoperator of the super-operator $[H,\cdot]$

Balarka Sen

@heather Gromov is impossible to read.

Semiclassical

and inasmuch as this standpoint goes counter to the hydrodynamics story, my gut feeling is against hydrodynamics

Anonymous

@EmilioPisanty $Q$ and $\Phi$ can describe the whole state of the RLC circuit at any point of time, isn't it? So, modulo some constant factors I think it is legitimate to consider them as canonically conjugate variables on a classical phase space

Anonymous

How to formally write it down, I'm not sure though

Anonymous

Intuitively it looks fine to me

Semiclassical

9:05 PM

a physical theory that allows non-local correlations is weird but consistent with reality. a physical theory that allows non-local signalling is a bridge too far for me

Emilio Pisanty

@Blue the heuristic models for superconductivity always looked dodgy to me

it goes a fair way downhill from just the canonical-conjugate-variable-ness

heather

@BalarkaSen basically, an equation with partial derivatives =)

Balarka Sen

correct

Emilio Pisanty

they have these weird manipulations where you add a source of current (I think?) and then it creates a linear potential, on top of which is a sinusoidal washboard coming from a Josephson junction, and then you use that to make this metastable qubit off of one of the wells?

vzn

@enumaris need to define some new concepts wrt "privileged rest frame" (a very old idea nearly 1 century). a "stretchable/ malleable" "spacetime fabric" is similar yet not quite the same.

heather

9:07 PM

but what is the point of the greek letter whose name i can't remember out front? does it just represent coefficients? @BalarkaSen

Emilio Pisanty

it looked awfully unkosher, but then again I was taught it by somebody who didn't do that for a living

heather

capital phi

Balarka Sen

@heather Ah, $\Phi$ (capital phi) is encoding the equation those partial derivatives of $f$ satisfy.

For example, consider the Laplace equation $\partial^2 f/\partial x_1^2 + \cdots + \partial^2 f/\partial x_n^2 = 0$.

heather

@BalarkaSen ah, so effectively the equation is like, we have an equation $\Phi$ that has partial derivatives of $f$ from order 1 to order $n$

Balarka Sen

Yes, correct!

Emilio Pisanty

9:08 PM

=( the late great Sean Barrett, in case @DanielSank has heard the name

heather

i see, the notation makes sense then.

but then...why set this equal to zero? Or does $\Phi$ only represent one "side" of the equation?

vzn

@BalarkaSen heard from Zee in here recently that gromov (may have?) worked on fluid dynamics some...? any interest? o_O :P

Jun 19 at 5:00, by Zee

Well , frankly I don’t blame them , since many people who work in the area don’t explore these deep connections. But these connections are there , for example fluid mechanics and Gromov H principle , or the work of vladmier Arnold or the theory of viscosity solutions in PDE theory

Balarka Sen

@heather That's correct.

Emilio Pisanty

@heather it's like saying that the equation $$y''(x) + y(x) = 0$$ has the form $$F(y(x),y'(x),y''(x)) = 0,$$ where (in that particular case) $F(a,b,c) = a+c$.

it's a way of keeping track of the structure of the equation

Balarka Sen

Emilio's example is good.

Emilio Pisanty

9:12 PM

in my example, it's used to rule out e.g. $y'''(x)+y'(x)=0$

heather

"rule out" - i.e., show immediately that that is not a term?

Balarka Sen

@vzn Not directly. The h-principle can be used to solve a family of equations which apparently come from fluid dynamics.

Emilio Pisanty

... not that @Balarka's example actually rules out all that much =P

vzn

@BalarkaSen heh ok had that feeling. (googling) ams.org/journals/bull/2012-49-03/S0273-0979-2012-01376-9/…

Emilio Pisanty

@heather exactly; the form $F(y,y',y'')=0$ forbids the presence of derivatives higher than the second order

heather

9:14 PM

@EmilioPisanty i see. okay, but then why would you use that notation in a case where nothing's really being ruled out?

Emilio Pisanty

it also rules out other possible ugly behaviours like, say, $y'(x) + y(x-1)=0$ or some such

Balarka Sen

@EmilioPisanty It does! My $\Phi = 0$ stops at the $r$-th order

Emilio Pisanty

@heather see my example above

Balarka Sen

It's an $r$-order partial differential equation

heather

@EmilioPisanty ah. yes, that does seem a nice thing to rule out.

okay, thank you, i think that makes sense then.

Balarka Sen

9:15 PM

Lol at that ninja edit.

Emilio Pisanty

@BalarkaSen I know, I know

I guess it does rule out that same nonlocal behaviour

i.e. that it is the same difference

@BalarkaSen added emphasis for additional clarity, nothing more ;-)

Balarka Sen

@heather Now that that's clear, the partial differential relation (PDR) corresponding to the PDE is obtained from replacing the derivatives of $f$ with derivatives of other totally unrelated functions. So $$\Phi(f, \partial g_i/\partial x_i, \partial^2 h_{ij}/\partial x_i \partial x_j, \cdots, \partial z_{i_1 i_2 \cdots i_r}/\partial x_{i_1} \cdots \partial x_{i_r}) = 0$$

Emilio Pisanty

@heather just for clarity, this is the point where a reasonable and unbiased observer can legitimately go

Balarka Sen

A solution to the PDR is a set of functions $f, g_i, h_{ij}, \cdots$ which satisfy this "algebraic" relation.

Emilio Pisanty

@BalarkaSen bejeeesus, that's horrifying

Balarka Sen

9:18 PM

lol

So for example, the partial differential relation corresponding to the partial differential equation $\partial^2 f/\partial x^2 + \partial^2 f/\partial y^2 = 0$ for a function $f = f(x, y)$ is the "algebraic(= non-analytic) equation" $\partial^2 f/\partial x^2 + \partial^2 g/\partial y^2 = 0$.

For two different functions $f = f(x, y)$ and $g = g(x, y)$

Therefore solutions to the PDR corresponding to the 2D Laplace equation is a pair of functions $(f, g)$ satisfying that relation of derivatives

heather

so...

that's a lot of notation

Balarka Sen

Mhm :) Sorry about that.

heather

let me see. so we substitute in a random new function $g$, but we do the derivatives with the same equation structure as in $f$, I assume that's why $f$ is still hanging out in your first equation?

Balarka Sen

"so we substitute in a random new function g, but we do the derivatives with the same equation structure as in [the original PDE]"

Well, the original $f$ is not a specific function, right?

It's a variable

$f$, $g$, etc are all variables which vary over functions

If you have a solution to the equation, it's specifying those variables as some definite functions.

heather

oh, right, because it's an equation relating functions, so functions are effectively variables. so then why is $f$ still there if we are replacing it with $g$?

Balarka Sen

9:27 PM

Sorry for that confusion. :) Since these are variables, I was lazy and didn't want to rename $f$ when I made the PDR out of the PDE.

Let me mend that.

Original PDE was "Given $\partial^2 f/\partial x^2 + \partial^2 f/\partial y^2 = 0$, solve for $f$"

PDR is "Given $\partial^2 g/\partial x^2 + \partial^2 h/\partial y^2 = 0$, solve for $(g, h)$"

@heather OK?

heather

okay, so we replace each partial derivative of whatever order of $f$ with a partial derivative of whatever order of some new function...a new function for each order, or just two new functions?

Balarka Sen

In this specific example. If you had some terrible PDE involving lots of derivative of the same function $f$, you'd replace each of those derivatives by derivative of a different function each time.

heather

gotcha.

so effectively a new function for each derivative.

Balarka Sen

Yup.

And that's what the PDR corresponding to the PDE is.

Just an abstract relation between the partial derivatives, not an equation. The name makes sense, kinda.

heather

let's see, the next line...why are there subscripts on $g$ and $h$ but not on $f$?

do $g$, $h$, and $f$ all represent solutions to the PDE? (how, if $g$ and $h$ are used only on a single derivative?)

Semiclassical

9:35 PM

I keep wanting "h-principle" to somehow be related to semiclassical methods in QM, if only because those formally arise via asymptotic series in Planck's constant (i.e. small $h$)

Balarka Sen

$f$ is the term corresponding to the zero-th derivative of in our original PDE.

Semiclassical

alas, that's not at all true as far as I know

heather

okay, so the subscripts indicate the derivative the function is associated with.

Balarka Sen

Yep.

heather

oh, so in, for example, a PDE like $f'' + g' + h$, the solution $f$ is the "acceleration solution", $g$ the "velocity solution", $h$ the "position solution"...but that doesn't really make sense. how can you find a solution here introducing multiple variables like that?

Balarka Sen

9:37 PM

Well, the PDE would be $f'' + f' + f = 0$. The PDR corresponding to that is what you said, $f'' + g' + h = 0$.

For each appearance of the $k$-order mix partial $\partial^k f/\partial x_{i_1} \cdots \partial x_{i_k}$ in our original PDE, replace that term with $\partial^k f_{i_1 i_2 \cdots i_k}/\partial x_{i_1} \cdots \partial x_{i_k}$ for some completely new function $f_{i_1 i_2 \cdots i_k}$

heather

but...why?

Balarka Sen

I'm getting there :)

heather

ah.

sorry =)

Balarka Sen

It's a good question

heather

let me ask a different question then. why can you treat both $g$ and $h$ as solutions?

Balarka Sen

9:39 PM

It's like a diophantine equation.

You have multiple variables

Solve for all of them

Of course there will be many many many many solutions

Solving the PDR is usually very easy. The Laplace equation $f'_x + f'_y = 0$ is "hard", but $g'_x + h'_y = 0$ is so easy: $(g, h) = (-x, y)$.

Lots of solutions come from free

heather

oh, i see

so effectively you're looking when solving the equation with $f$ for a PDR solution where all the "individual" solutions are equal.

Balarka Sen

Yes! That's when a solution to the PDR would give me a solution to the PDE

heather

ah. that almost sounds like it could be really useful if you're trying to numerically solve something.

if you can easily find the PDR solutions, you find a bunch of them until you find the ones that "match".

Balarka Sen

That was the key idea of h-principles!

heather

of course, if you have a large solution set for the PDR, that could take a while.

Balarka Sen

9:42 PM

You have discovered h-principles independently of Gromov

heather

with a lot of help from you =P

but i assume there's a formal notation for h-principles. what sorts of boundaries are there on this? does it work for anything?

danielunderwood

@EmilioPisanty Is there a name for that type of equation ($y'(x) + y(x-1) = 0$ or similar with offset independent variables)? I've never seen such a creature and it looks interesting

Semiclassical

Delay differential equation (regarding x as “time”)

Balarka Sen

@heather All good questions! Let me lay that out a little formally.

heather

(and another question, how can you tell how large the solution space is for the PDR is?)

@BalarkaSen cool, thank you.

Balarka Sen

9:44 PM

You're asking great questions after great questions

Let me answer one by one

Suppose $\Phi = 0$ is a PDR coming from a PDE. It's said to satisfy the the $h$-principle (short for homotopy principle) if any solution $(f, g_i, h_{ij}, \cdots)$ of the PDR is $\epsilon$-close to a solution $F$ to the original PDE.

By which I mean, $|f - F| < \epsilon$, $|g_i - \partial F/\partial x_i| < \epsilon$, $|h_{ij} - \partial^2 F/\partial x_i \partial x_j| < \epsilon$, etc etc.

Semiclassical

@danielunderwood the usual way I know to solve a delay differential equation like that is to take the Laplace transform of the equation

Balarka Sen

What this means is that once you have solved the PDR (easy, like we discussed; lots of solutions come for free), you can find an actual solution to the PDE within an $\epsilon$-vicinity of that.

heather

so you're saying if the solutions to the PDR are close to a solution $F$...does this mean that the solutions are also close to each other?

and also, how can you tell if the PDR solution(s) are within $\epsilon$ of $F$?

danielunderwood

At least a few I saw had some pretty ugly looking solutions. Do they pop up in physics anywhere? They kind of remind me of retarded potentials, but I think everything I've seen there was inside of an integral

heather

(also, if my first comment above is true, how many of the solutions must be close to each other?)

Balarka Sen

9:50 PM

I haven't stated anything beyond the meaning of "h-principle" yet. It says precisely that within a small vicinity of your PDR solution you can find some PDE solution

It's the definition of the "h principle". I haven't told you when it holds.

heather

oh, sorry, reading too much into it =P it basically confirms that if you look at PDR solutions you can find a PDE solution.

Balarka Sen

Correct!

It's the most optimistic hope in that direction, stated formally.

heather

gotcha.

Balarka Sen

Gromov proved in his PhD thesis that the $h$-principle always holds for partial differential (non-strict) inequalities

Danu

@ConstantineBlack I did a BSc. in physics and am now doing a PhD in pure math (differential geometry)---what do you want to hear about?

Balarka Sen

9:52 PM

(More or less)

Danu

Hi Balarka

Balarka Sen

Hi @Danu

Semiclassical

@danielunderwood not so much in Physics I think

heather

@BalarkaSen so...what about equations?

Semiclassical

But in biology they show up

Danu

9:53 PM

You're learning about h principles? cool

heather

@Danu yeah, Balarka got me interested =)

they seem really useful.

Danu

hmm :P

heather

@Danu he hasn't told me the limitations yet =P

Danu

I don't really know,but suffice it to say I don't see anyone using them outside like symplectic/contact topology

But super cool math!

Worth learning just as math

Balarka Sen

@heather $h$-principle doesn't necessarily hold for partial differential equations! The thing is that those are closed equations. If you have a PDE, and a solution $f$ to the PDE, and if you wiggle $f$ to a function $g$ a little so that the difference $\|f - g\|$ is realy small, then $g$ might not be a solution to the original PDE anymore.

heather

9:54 PM

@Danu oh, really? i mean, that explains why it's called the homotopy principle.

Semiclassical

@danielunderwood the opening paragraph of the Wikipedia page has some pertinent remarks as to their applications : en.m.wikipedia.org/wiki/Delay_differential_equation

Balarka Sen

But this is of course always true for open PDI (partial differential inequalities)'s, because wiggling something to be inside of a region cut out by inequalities always stays inside that region

Emilio Pisanty

Delay differential equation

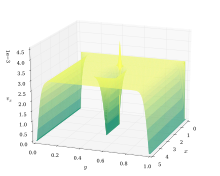
In mathematics, delay differential equations (DDEs) are a type of differential equation in which the derivative of the unknown function at a certain time is given in terms of the values of the function at previous times. DDEs are also called time-delay systems, systems with aftereffect or dead-time, hereditary systems, equations with deviating argument, or differential-difference equations. They belong to the class of systems with the functional state, i.e. partial differential equations (PDEs) which are infinite dimensional, as opposed to ordinary differential equations (ODEs) having a finite...

heather

@BalarkaSen so if inequalities work, but equations don't, why can't you use it for equations by effectively tightening inequalities around the solution to the equation? (if that makes any sense)

Emilio Pisanty

duh, just saw the answer below your comment

heather

9:56 PM

i guess we don't know the solution, so we don't know what to "tighten" around...

Balarka Sen

You're surprisingly close to the heart of the idea, @heather.

Danu

So Balarka, ya know where you're going to uni? :)

Balarka Sen

Gromov proved that $h$-principles hold for a large class of partial differential equations as well, to everypone's surprise. Those are called "ample" differential equations. The solution procedure goes exactly the way you state, by approximating actual solutions of the PDE by a Cauchy sequence of solutions of the PDR, and one has to do careful analysis to show that the limit converges

heather

@BalarkaSen here, i'm sorry, but is $g$ not a solution anymore because of the small difference, or because $f$ is wiggled, or...? i lost track of this one =)

Balarka Sen

@heather $g$ is not a solution anymore because of that small error between $g$ and $f$, however small, yep.

It's an almost-solution.

heather

9:58 PM

@BalarkaSen gotcha, okay. thanks for clarifying.

Balarka Sen

@Danu More or less.

heather

but then...why can't you look at what all the different PDR solutions $g, h, ...$ "approach"?

Balarka Sen

The limit will not in general converge :)