In matrix calculus, Jacobi's formula expresses the derivative of the determinant of a matrix A in terms of the adjugate of A and the derivative of A. If A is a differentiable map from the real numbers to n × n matrices,
Equivalently, if dA stands for the differential of A, the formula is
It is named after the mathematician C.G.J. Jacobi.
== Derivation ==
We first prove a preliminary lemma:
Lemma. Let A and B be a pair of square matrices of the same dimension n. Then
Proof. The product AB of the pair of matrices has components
Replacing the matrix A by its transpose AT is equivalent to permuting...
If $1-ab$ is invertible for $a$, $b$ in a (noncommutative) ring then so is $1-ba$.
Proof:
$$(1-ba)^{-1} = 1+ba +baba+\cdots = 1+b(1+ab+abab+\cdots)a = 1+b(1-ab)^{-1}a,$$
The meaningless infinite series give the right answer (which is hard to guess).
In mathematics, the real projective plane is an example of a compact non-orientable two-dimensional manifold, that is, a one-sided surface. It cannot be embedded in standard three-dimensional space without intersecting itself. It has basic applications to geometry, since the common construction of the real projective plane is as the space of lines in R3 passing through the origin.
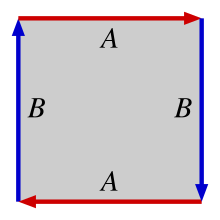
The plane is also often described topologically, in terms of a construction based on the Möbius strip: if one could glue the (single) edge of the Möbius strip to itself in the correct direction, one would obtain t...
