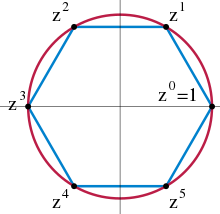
00:00 - 16:0016:00 - 23:00
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
user147690
00:00 - 16:0016:00 - 23:00