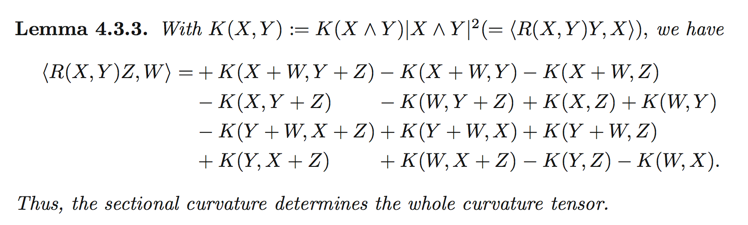I have this $2^n*2^n$ matrix that represent the evolution of a system of $n$ spin.
I know that I can have only one excited spin in my configuration a time.
(eg: 0110 nor 0101 ar not permitted, but 0100 it is)
$s_+$ is defined with $s_x+is_y$ and $s_-$ is defined with $s_x-is_y$
(creation and a...
@Qmechanic Hm...not unclear, I'd say, but the comment by MichaelBrown just answers it - it's probably not interesting enough a question that anybody cared to write that up into an answer.
@ACuriousMind If I have a manifold with vanishing sectional curvature everywhere the exponential map is automatically an isometry because the manifold has to be Euclidean, right?
@Huy @skullpetrol I finally figured out that proof for the circle and the Gaussian curvature. It turns out there's a very helpful Taylor series for the length of a Jacobi field, which has the Gaussian curvature as the third order coefficient.
@0celo7 I'm structuring what I'll teach when I do complex numbers. anything else absolutely necessary apart from eulers formula/polar form and the fundamental theorem?
hi guys! under an "infinitesimal" translation $x^\mu\mapsto x^\mu-a^\mu$, why does the Lagrangian density transform as $\mathcal L\mapsto\mathcal L+a^\nu\partial_\mu({\delta^\mu}_\nu\mathcal L)$?
I'm a bit confused about Noether's theorem (or about calculus of variations in general) when it comes to the translational symmetry $x^\mu\mapsto {x'}^\mu=x^\mu-a^\mu$. My professor just wrote that if the Lagrangian density depends on $\phi$ and $\partial_\mu\phi$ only, then it transforms like
$...
@Bass He is trying to say that if the function $f(x,y,y')$ is independent of $x$ then not only do we have that $f(x + a,y,y') = f(x,y,y')$ but since $f(x + a) = f(x) + (\frac{d}{d x}f(x)) a$ we have $f(x) = f(x) + (\frac{d}{d x}f(x)) a$
Anybody have a nice hand-wavey gooey-feely way to remember that $a \approx \sqrt{\omega} x + i \frac{1}{\sqrt{\omega}} p$? i.e. why does $\sqrt{\omega}$ make position unitless or $\frac{1}{\sqrt{\omega}}$ make momentum unitless?
@bolbteppa not sure about your notation, if $f$ is independent of $x$, then $\frac{d}{dx}f=0$. Btw I know what a Taylor expansion is, but I'm having trouble anyways, see the linked PSE question..
@bolbteppa I'm not sure, but if you put $a=\sqrt{\frac{m}{2\hbar}}(\hat x+\frac{i}{m\omega}\hat p)$, you should get a dimensionless number operator $\hat N=a^\dagger a$.
so in your definition you seem to have $m=1$ and $\hbar=1$
@bolbteppa aarrgh of course! I was just confused by the $\delta_\nu^\mu$ in the last step of $\mathcal L(x+a)\approx\mathcal L(x)+a^\mu\partial_\mu\mathcal L=\mathcal L(x)+a^\nu\partial_\mu(\delta^\mu_\nu\mathcal L)$. Thanks!
@Bass Lets look at $\mathcal{L} = \mathcal{L}(x^{\mu},\phi,\partial_{\nu}\phi)$ in an action functional via an example with easier notation, e.g. $$S_1 = \int_0^1 \int_0^1 \mathcal{L}_1 dx dy = \int_0^1 \int_0^1 \mathcal{L}_1(x,y;z;\partial_x z, \partial_y z)dxdy = \int_0^1 \int_0^1 (x^2 + y^2 + z^2 + (\partial_x z)^2 + (\partial_y z)^2)dxdy$$ we have $z$ playing the role of $\phi$. So what are we doing?
We are minimizing $S$, giving us a PDE which we solve to find the surface $z = z(x,y)$ minimizing this functional. Clearly this example is not invariant under $x \rightarrow x^* = x + \varepsilon$ right? However $S_2 = \int \mathcal{L}_2(x,y;z;\partial_x z, \partial_y z) = \int z^2 + (\partial_x z)^2 + (\partial_y z)^2$ would be invariant.
In other words, $S_1(x^*,y;z;...) \neq S_1(x,y;z;...)$ but $S_2(x^*,y;...) = S_2(x,y;...)$. Now Taylor expand both cases and see what happens, there is a difference...
@Danu Yeah, thought so, but I think there is no way to break that down. There's a large work of the connection between supersymmetry and Morse theory, and of a non-relativistic supersymmetric QFT to Floer theory behind that SYM theory that finally gives the Donaldson invariants, and in fact Witten's and Atiyah's papers are already comparatively lucid. I have to say I cannot compess that into much less than what is written already.
As I see it, it's three rather long papers: Witten's "Supersymmetry and Morse theory", then Atiyah's "New invariants of three- and four-manifolds" (or somehting like that) and then Witten's "Topological Quantum Field Theory" that tell the story, and they all heavily rely on the reader to be familiar with both QM and QFT, and some basic supersymmetry applications.
This might be where the Q&A format hits the boundary of what it is suitable for - perhaps a series of smaller questions could break this up into somthing more digestible.
@ACuriousMind Seriously...since the Riemann curvature is determined completely by the sectional curvature, then vanishing sectional curvature implies the manifold is Euclidean.
@Danu I can never settle on which audience to write for, and end up writing next to nothing, leaving several less-than-half-finished drafts in the limbo...
@Danu I...had a few lazy months lately. My new year's resolution is to get a master's thesis on gauge theories, conformal field theories and/or moduli spaces, there are some good options here.
1. I'm really getting into mathematics---topological & geometrical things (low-dimensional, let's say $n\leq 4$). I'd like to have a thesis that relates to these things.
2. I do not want to be "pushed into a corner" too much by my thesis (topic or supervisor). This rules out things like pure math, but possibly also string theory.
3. I want to have a supervisor that is at least somewhat approachable, but also one that is somewhat well-known. That rules out the really really famous ones like Dvali (too busy), but also makes me doubt about some others.
That's it.
also 4. (part of 2.) I would like to have some kind of a topic that is at least in a general direction that seems somewhat promising (again, problematic for string theory).
Do any of you folks know of a book or other reference which explains, in serious detail, the link between a stochastic process and the statistics of analysis made on a realization (i.e. measurement) of that process?
I, personally, would throw your considerations 2. and 4. right out - do what you want. I can understand 3., but again, I'd rate it higher to find someone you're comfortable with than someone "well-known".
@DanielSank I think that in the current state of theoretical physics, "promising" is a very subjective assessement, so I do not put much stock in it. Of course you shouldn't go off and do something no one thinks is a good idea to begin with, but...I trust Danu to not want to do such things in the first place ;)
@DanielSank I think the largeness of e.g. the strings community it not necessarily the most important thing to think about in connection to the point I raised.
The problem is rather that it seems there is a recent trend to not be so happy with strings because of the failure of LHC to reveal anything stringy.
@Danu Then...I'll manage. Or not, I don't know, I never had to deal with serious failure so far. But I'm not sure that I'd want a job to do research on things I don't consider interesting.
@ACuriousMind A significant reason is also that you get to use your brain to solve hard problems without worrying about keeping your job for more than two years.
How do I find my answers to questions? I see no way to search for them or anything I wrote. And, why do I have to even ask this question?
