Problem Solving Strategies

kyle campbell

03:56

@JohnRennie immediately after the switch is closed, is the current through R1 and R2 just zero?

my reasoning is that the current through the inductor is zero and is in series with R2 so the current through R2 is zero and since R1 is in parallel with R2 and the inductor, R1 should also be zero

I'm ignoring transients for now, I just mean the instant after the switch is closed

John Rennie

@kylecampbell immediately after closing the switch the current through $R_2$ is indeed zero, but the current through $R_1$ is just the usual $I = V/R = 30/R_1$

kyle campbell

@JohnRennie why? isn't R1 in parallel with the circuit elements R2 and inductor?

is there a voltage drop across one of them even though the current is zero through them?

John Rennie

If two components A and B are in parallel then the voltage across them is the same, $V_a= V_b$, and the total current is the sum of the two currents, $I = I_a + I_b$.

kyle campbell

okay, but if the current through the resistor R2 is zero then where's the voltage dropping across the right arm of the circuit?

the inductor?

John Rennie

Yes, the voltage is dropped across the inductor. Immediately after the switch us closed it behaves like a very large resistor.

kyle campbell

04:03

ah I see

Nobody recognizeable

@JohnRennie hi are you done with @kylecampbell?

kyle campbell

yes, have at 'er

Nobody recognizeable

@JohnRennie I am sorry I pinged you twice. Can you switch back to pss?

@JohnRennie I was asking what would happen if a high frequency current flows through conductor? Why doesn't it conduct the current?

John Rennie

@Nobodyrecognizeable For a regular conductor like copper nothing special happens as you increase the frequency.

user8718165

Good morning sir @JohnRennie

Nobody recognizeable

04:11

@JohnRennie more specifically they are asking "we can't transmit em wave having frequency in GHz using copper wire" explain.

John Rennie

@Nobodyrecognizeable My computer runs at 3GHz and it transmits signals of that speed through copper just fine.

At work we use 10GHz network cables made from copper.

Nobody recognizeable

@JohnRennie I see. Maybe a garbage question. Anyway can I ask another?

John Rennie

@Nobodyrecognizeable well where did you see this? Maybe they are talking about some special situation?

Was it an exam question?

Nobody recognizeable

@JohnRennie yep. University exam paper actually.

John Rennie

You do have to be careful with the cable design at these high speeds because the cables act as aerials i.e. they produce EM radiation.

That can cause power loss.

Nobody recognizeable

04:15

@JohnRennie all right. Thanks for the hint. Can I ask another problem?

John Rennie

Typically cables that have to carry these frequencies are designed to minimise the energy lost as radiation e.g. by using twisted pairs.

Twisted pair

Twisted pair cabling is a type of wiring in which two conductors of a single circuit are twisted together for the purposes of improving electromagnetic compatibility. Compared to a single conductor or an untwisted balanced pair, a twisted pair reduces electromagnetic radiation from the pair and crosstalk between neighboring pairs and improves rejection of external electromagnetic interference. It was invented by Alexander Graham Bell. == Explanation == In a balanced line, the two wires carry equal and opposite signals, and the destination detects the difference between the two. This is known as...

@Nobodyrecognizeable yes, you're welcome to ask.

Nobody recognizeable

@JohnRennie when you have a circuit of many inductor, capacitor and resistances in various loops then how do you solve for current? Ie general equation of current as a function of time?

John Rennie

@Nobodyrecognizeable I don't think there is a simple answer to that. You look at the circuit and see what is in parallel and what is in series.

For combinations of R, C and L you use phasor diagrams.

Any questions you encounter are unlikely to be too complicated. After all you have to solve them in a few minutes.

Nobody recognizeable

@JohnRennie uploading a circuit.

@JohnRennie take this and have an ac source in left loop ac source as E=E_0sinwt.

@JohnRennie if you apply kvl then you'll have three variables. How to eliminate them?

John Rennie

I've got a problem at work I need to deal with.I'll be a few minutes.

That circuit looks complicated. Did you get that set as a problem or is it one you made up?

Nobody recognizeable

04:30

@JohnRennie honestly I faced a problem like this.

@JohnRennie when you come back please ping me.

John Rennie

@Nobodyrecognizeable that's more complicated than I know how to deal with. I would probably use complex impedance, but the algebra would quickly get very complicated.

user8718165

04:50

@JohnRennie hello sir

John Rennie

@user8718165 morning :-)

@Nobodyrecognizeable hi

@Nobodyrecognizeable if you redraw the circuit like this you can see that R2, C2, L2 and R3 from a unit. If we call the combined impedance impedance of this Z1 then our circuit is just L1, C1, Z1 and R1 in series and you can do that with a phasor diagram. So we just have to calculate what Z1 is.

C2, L2 and R3 are in series so we can use a phasor diagram for them, call the combined impedance Z2, then we have R2 and Z2 in parallel.

user8718165

05:30

@JohnRennie sir can you please help me with the calculation? :-)

John Rennie

@user8718165 which calculation?

user8718165

@JohnRennie sir this one

John Rennie

@user8718165 OK, suppose we have a 1m cube i.e. the sides are 1m. We add an external pressure of 1 atm and the sides decrease to $1-x$ m, where we have to figure out what $x$ is.

So the volume has decreased to $(1-x)^3$. OK so far?

user8718165

@JohnRennie all the sides decrease in size

John Rennie

@user8718165 yes. The pressure is uniform around the cube so the whole cube compresses uniformly i.e. it stays cubic.

user8718165

05:38

@JohnRennie if the length and breadth of the water block become short....they won't be touching the vessel...not getting it

John Rennie

There are two different questions here:

1. if we consider a cubical volume of water somewhere in the vessel what happens to that cubical volume?

2. If we consider the whole vessel, and assume the width of the vessel doesn't change, what happens to the depth of water in the vessel?

user8718165

@JohnRennie okay

John Rennie

I suspect I'm thinking about question 1 while you're thinking about question 2.

user8718165

@JohnRennie aha...got it...you aren't concerned about the bounds of the vessel right? You're applying pascal's law

for qn1

John Rennie

Yes

user8718165

05:43

@JohnRennie okay sir...got it...we're answering different questions....XD

Nobody recognizeable

@JohnRennie sorry...

@JohnRennie Thanks.

@JohnRennie I recall the question was actually with dc sourse. So sorry. I don't know if there is a way to eliminate from 2 or more networks in dc circuit.

John Rennie

@Nobodyrecognizeable ah, OK. Presumably it was a question like what are the currents immediately after the switch is closed, and what are the charges on the capacitors after a long time?

Nobody recognizeable

05:59

@JohnRennie can we deduce the equation of charge as a function of time ie as in an simple RC circuits. I think this will be a question of coupled oscillators. I'm just running short of equations to determine the constants. And we don't have the facility to use complex exponentials as well. And you have q1, i1, di1/dt as well as q2, i2, di2/dt as well.

John Rennie

@Nobodyrecognizeable You can, but it's going to be complicated.

Nobody recognizeable

@JohnRennie after long I think the capacitances will be fully using the voltage as they will charge themselves and offer infinite resistances.

@JohnRennie I am just too eager to do that! Do you have enough time?

John Rennie

@Nobodyrecognizeable after a long time the charge on C2 will be zero because it discharges through R2. The voltage across C1 will be the supply voltage .

@Nobodyrecognizeable I don't have time to do the full calculation. Sorry, but it will takes ages.

Nobody recognizeable

@JohnRennie all right. No problem.

kyle campbell

@JohnRennie I've got a couple q's

Nobody recognizeable

06:06

@JohnRennie BTW I think we can't do that as we don't have enough equations to eliminate the constants.

John Rennie

@kylecampbell hi

kyle campbell

(whenever you guys are done)

John Rennie

@kylecampbell I think we're done

Nobody recognizeable

@JohnRennie Thanks. Have a nice day.

kyle campbell

@JohnRennie so the question states that the circuit reached a steady state, and then you flip the switch to position 2

now, I said that the potential difference across the inductor immediately after flipping the switch to 2 is zero. my reasoning for this is because before (at 1) it was zero, and the potential difference across an inductor should be continuous (I'm assuming this follows from the fact that the current should be continuous)

but that's not right

I don't know what's wrong with my reasoning though

John Rennie

06:10

Flipping the switch is a discontinuous event, so you can't make arguments based on continuity.

kyle campbell

oh

so how do I reason questions like this out then?

John Rennie

But what you can say is that an inductor resists changes to the current flowing through it. Before the switch is flipped the current though the inductor is 2A. Yes?

kyle campbell

yes

John Rennie

Physically what happens is that the current through the inductor builds up a magnetic field around the inductor, and that field stores energy. When you flip the switch that field starts to collapse and the energy stored in the field goes into driving the current round the circuit.

kyle campbell

so the inductor starts behaving like a battery to the resistors?

as it discharges

John Rennie

06:13

Yes. Immediately after flipping the switch the inductor continues to push a 2A current through the 16 ohm resistor.

kyle campbell

in the same direction as before?

as in, does the current direction switch to cw or does it stay ccw around the (now smaller) loop?

John Rennie

Yes, the current through the inductor has the same direction and magnitude. So the current in the inductor is flowing downwards and the current through the 16 ohm resistor flows upwards.

kyle campbell

interesting, so you can just find the potential difference by treating it like a power source in series with two resistors

John Rennie

Yes, the current is 2A so the voltages across the two resistors are 16 x 2 and 12 x 2.

And your equation for the inductor is $V = -L dI/dt$

kyle campbell

how do I find dI/dt?

oh but we can just use loop rule though

?

John Rennie

06:21

I have to deal with a problem at work. I'll be a few minutes, but hopefully only a few minutes.

kyle campbell

for sure

Jasmine

Hello please help me with the 11th one

kyle campbell

@JohnRennie one more question when you get back: if you have an emf source with $\epsilon = 100cos(8000t) V$, a resistor with $R = 100 \Omega$, an inductor with $L = 1.00 \times 10^{-3} mH$, and a capacitor with $C = 1.00 \times 10^{-9}$... how would you find the voltage across the inductor when the voltage across the resistor is zero?

John Rennie

@kylecampbell I'm back

kyle campbell

hi

John Rennie

06:30

The inductor equation is $E = -L dI/td$ and $E = IR$, where $R$ is the total resistance - $R = 28$ ohms.

If we rearrange this we get:

$$ \frac{dI}{dt} = -\frac{R}{L} I $$

And this is going to give an exponential decay or the current:

$$ I(t) = I_0 e^{-(R/L)t} $$

kyle campbell

right

John Rennie

And we know the initial current is $I_0 = 2$ amps.

@Jasmine it's going to look like a dipole field I think.

@Jasmine like this?

kyle campbell

I figured the other one out. Thanks again @JohnRennie

John Rennie

@kylecampbell cool :-)

@kylecampbell you drew a phasor diagram and figured out the phase difference?

kyle campbell

06:45

yes

Aladdin

@JohnRennie hello

John Rennie

@Aladdin hi

Aladdin

Can we try Q11

I am stuck at binomial simplification.....

John Rennie

Q11 of Jasmine's problem?

Aladdin

The dipole moment along P will be 2dsinθ

@JohnRennie yes

We can work sin θ from the diagram

John Rennie

06:59

You don't need to do anything complicated. For a single dipole the field marked in red at P is just $E = kp/y^3$, and the two dipoles will add to give a total field $E = 2kp/y^3$ where $p = QL$.

Aladdin

Aha yes. I am getting 2 as an answer. Don't think it's correct

John Rennie

The question says if the field is $nkQL/y^m$ what is $n+m$. The values of $n$ and $m$ are 2 and 3 so the answer is 5.

Aladdin

@JohnRennie but the point. Is not on the perpendicular bisector but at an angle

John Rennie

We are told that $y \gg L$ so we can ignore the fact the dipoles have a non-zero length and separation.

i.e. the four charges appear as a single dipole $p = 2QL$.

Aladdin

Nvm I get 5 too

@JohnRennie okay

So we can also assume the point lies on the perpendicular bisector of the dipole also from that

@JohnRennie hi. Are you still here

John Rennie

07:16

@Aladdin hi

@Aladdin from the symmetry the field at P is parallel to both lines $Q \to -Q$

Aladdin

Yes

Jasmine

@JohnRennie is the dipole you have shown in the diagram only for one pair of Q,-Q

John Rennie

@Jasmine the two pairs create a field in the same direction at the point P. The red arrow I've drawn is the sum of those two fields from the two dipoles.

The field from each pair would be in the same direction as the red arrow but half the size.

Jasmine

@JohnRennie ok

@JohnRennie why p=QL

John Rennie

07:32

@Jasmine that's the definition of a dipole moment. It's the charge times the distance between the charges.

Jasmine

To be honest I am not able to understand

John Rennie

If you two charges $+q$ and $-q$ separated by a distance $d$ then the dipole moment associated with the two charges is $p = qd$.

Jasmine

@JohnRennie I thought of the pair of charges are Q,-Q on the diagonals

John Rennie

Maybe I've misinterpreted the question, but it says the charges are Q, Q, -Q, -Q in cyclic order. I assumed that meant if we start at one corner and go round the square the charges are +Q then +Q then -Q then -Q i.e. the two +Q charges are next to each other, and likewise for the two -Q charges.

Like that

That seems to fit because the answer they give is what you would expect for the two dipoles.

Jasmine

@JohnRennie okay I understand now because of the given condition y>>L you have used the pair Q and -Q as a dipole

John Rennie

07:38

@Jasmine yes, exactly!

Jasmine

I had tried this question with too much use of trigonometry in it and used binomial theorem which even gave the wrong answer

John Rennie

Like that

You should be able to do it by doing the detailed calculation, but it's going to be a tortuous calculation.

Jasmine

@JohnRennie Thank you I got it !! :-)

John Rennie

We can go through it if you want.

Jasmine

@JohnRennie yes I actually want to go through it as I dont know what mistake I am making

John Rennie

07:43

Let me update my diagram ...

Jasmine

There two pairs of Q,-Q on the diagonals the net electric field will be in the sirection of -Q parallel to the diagonal for one pair

And for the other pair Q,-Q on the other diagonal the net electric will be again in the direction towards -Q parallel to the diagonal

And diagonals of square bisect at 90° and since both electric field are equal in magnitude so net will be $\sqrt{2}E_{net}$

John Rennie

I would do the calculation like this ...

The square base and the point P form a square based pyramid. Start by considering one of the faces as I've drawn on the right.

The distance $r$ will be a function of $y$ and $L$, but we don't need to worry exactly what the function is for now.

The field at P can be split into a component parallel to the line $Q$ to $-Q$ and a component perpendicular.

But the perpendicular component will be zero because the $+Q$ and $-Q$ charges will create equal and opposite perpendicular components and they will cancel.

Jasmine

@JohnRennie yes

John Rennie

So we only need consider the component parallel to the $Q$ to $-Q$ line.

OK so far?

Jasmine

@JohnRennie yes

John Rennie

07:55

And when we do the other two charges, the back face of the pyramid, they will also cancel the perpendicular component and they will produce a parallel component in the same direction. So the total field will be parallel to the two $Q$ to $-Q$ lines.

So we can do the calculation for just one face, as drawn, then double it to get the final result.

Is this all OK? If so we can start actually calculating something!

Jasmine

@JohnRennie yes

John Rennie

OK. Start with the left diagonal. The field is along the diagonal with magnitude $kQ/r^2$.

Jasmine

@JohnRennie yes

John Rennie

Call the half angle at the top $\theta$ (so the angle between the two diagonals is $2\theta$) then the horizontal component of the field is $E\sin\theta = kQ\sin\theta/r^2$

Where $\sin\theta = (L/2)/r$

OK so far?

Jasmine

@JohnRennie ok

John Rennie

08:01

Substitute for $\sin\theta$ in our expression and we get:

$$ E = \frac{kQL}{2r^3} $$

Jasmine

@JohnRennie yes

John Rennie

That's the field for the $+Q$ charge. From the symmetry the field due to the $-Q$ charge is going to be the same, so the total field from both the charges is:

$$ E = \frac{kQL}{r^3} $$

Jasmine

@JohnRennie yes

John Rennie

Note that $QL$ is just the dipole moment $p$, so we have got the standard equation for the dipole field $E = kp/r^3$. That's the equation I started with when I was discussing the problem with Aladdin.

And the other two charges give the same result, so the total field for all four charges is:

$$ E = \frac{2kQL}{r^3} $$

Now we need to express $r$ as a function of $y$ and $L$.

But it should be obvious that if $y \gg L$ then $r \approx y$ and we don't really need to bother. But I will go through it if you want.

Jasmine

@JohnRennie okay

John Rennie

08:08

So our final result is:

$$ E \approx \frac{2kQL}{y^3} $$

Jasmine

@JohnRennie yes....

I got it :-)

John Rennie

Note that I haven't cheated anywhere in the calculation, but I have taken advantage of the symmetry to simplify it as much as possible.

With complicated calculations it's always worth looking hard to see if they can be simplified.

Or of course you could do what I did first, wave your hands in the air and say "oh look it's just two dipoles" :-)

2

Jasmine

@JohnRennie yes,, I have asked for the other way because the the other question just asks me to find Electric field on the centroid of an equilateral triangle at a distance y above it without any condition given

I have got the correct answer for both the questions :-)

John Rennie

Cool :-)

Aladdin

08:36

@JohnRennie hello. Are you free

John Rennie

@Aladdin hi, yes I'm free.

Aladdin

@JohnRennie in Q28 why is the answer not zero

The flux for the cylinder is zero, right

John Rennie

It's another infinite charged plane question.

With an infinite charged plane the field looks like this (diagram incoming):

So just like the question says the field points in opposite directions on the two sides of the plane.

Aladdin

Yes

John Rennie

Your cylinder is like this, so the charge inside the cylinder is the charge on the disk shown by the dashed lines.

Aladdin

08:47

Okay

John Rennie

i.e. if the cylinder radius is $r$ then the charge is $\sigma \pi r^2$, where $\sigma$ is the area charge density on the infinite plane.

Ah, OK, the question tells you the area $\pi r^2 = 100 m^2$

So you need to work out $\sigma$ from the field.

Actually, just use Gauss' theorem using the ends of the cylinder as your Gaussian surface.

Aladdin

Ok flux will add because both of them are going out of cylinder

I was Substractimg them

John Rennie

Oops :-)

Aladdin

I get the answer now

@JohnRennie though I searched the internet and found the intersection of plane and cylinder is coming ellipse

John Rennie

@Aladdin If the axis of the cylinder is not normal to the plane then the intersection will be elliptical.

A circle is of course a special case of an ellipse where the major and minor axes are equal.

Aladdin

08:57

So what is thr intersection here..

John Rennie

We get a circle for the intersection if and only if the axis of the cylinder is normal to the plane.

@Aladdin is the axis of the cylinder normal to the plane?

Aladdin

@JohnRennie it's not specified in there so I guess not

John Rennie

@Aladdin the question says: the axis of the cylinder is along X-axis, and it also tells you the field is in the positive and negative $\hat i$ direction i.e. the field is also along the $x$ axis. So the axis of the cylinder is parallel to the field. Yes?

Aladdin

Yea. I can see now how intersection is circle

John Rennie

Cool :-)

Aladdin

09:07

@JohnRennie can you try Q34

I did : 0.06=qEd

Here d is the distance where it stops

It should be 2qed

John Rennie

Let's draw a diagram:

Aladdin

In the end I get quadratic but no answer matches

I think this is simple harmonic motion too

John Rennie

The charge stops when the PE change is equal to the initial KE of 0.06J. Yes?

Aladdin

Yes

John Rennie

At a distance $d$ the PE change is $\Delta U = 2kQ^2/3 - 2kQ^2/r$

Where $r = \sqrt{9 + d^2}$

@Aladdin OK so far?

Aladdin

09:19

Okay. I think I got my mistake

John Rennie

You could solve this equation for $d$, but it's probably quicker to just try each of the four answers and see which fits.

Aladdin

Electric field would change along the, pathso qEd was wrong

John Rennie

@Aladdin yes

Aladdin

@JohnRennie a dipole is aligned to the electric field. Where would it experience a force towards

Here force = torque?

John Rennie

@Aladdin If the dipole is aligned with the field there is no net force or torque on it.

Aladdin

09:33

If it's slightly at an angle with the electric field then the force will be in directon of torque

John Rennie

Draw the dipole as a pair of charges at an angle to the field and draw in the forces. This will make it obvious why there is a torque. I can draw the diagram if you want.

Aladdin

Yes it's obvious why there is torque. Let me post the question for more clarity

How to rotate?

John Rennie

Give me a moment to flip your image the right way up ...

@Aladdin Which question?

Aladdin

51

I think it should be no force..

John Rennie

The numbers aren't shown on your picture ...

The middle question?

@Aladdin that one?

Aladdin

09:45

Yes

John Rennie

Note that the field lines are not parallel.

At least I assume they aren't parallel and it's not just the way the picture was taken

The field lines spread out from left to right, so the field gets weaker as we move from left to right. Yes?

Aladdin

Yes

John Rennie

So the force on the -q charge is $F_- = -qE_1$ and the force on the +q charge is $F_+ = +qE_2$ where $E_1 > E_2$.

It looks from the diagram as if the dipole is aligned with the field, so the forces are in the same direction and we simply add them to get the total force.

@Aladdin can you take it from here?

Aladdin

Yes

I think C

John Rennie

Yes, the dipole will move left.

Aladdin

10:11

@JohnRennie hi

In an electric field the potential at a point given by the following relation V=343/r.The electric field at r=3i+2j+6k is

I don't have any idea what to do here

John Rennie

Is that:

$$ V(r) = \frac{343}{r} $$

Aladdin

yes

John Rennie

There are two ways to approach this. The first is to spot that this is just the potential for a point charge $V(r) = kQ/r$ so the field is just the field due to a point charge.

Aladdin

Ok

John Rennie

So if you put your point charge at the origin you just need to calculate the field at the point (x=3, y=2, z=6)

So $r^2 = 3^2 + 2^2 + 6^2$, use that to calculate $E = kQ/r^2$ then calculate the x, y and z components.

Aladdin

10:18

That makes sense

I never thought of this way

John Rennie

That involves a bit of staring at 3D diagrams trying to decide what angle to take the sine or cosine of :-)

Aladdin

What's the other way

John Rennie

The alternative is that $E = -\nabla V$

Where $\nabla = (d/dx, d/dy, d/dz)$

Aladdin

Okay. How to use this

John Rennie

This looks scary at first glance because you've got this weird new operator $\nabla$, but it's actually really simple.

The potential $V$ is a scalar i.e. just a function of $r$ and it doesn't have a direction.

Aladdin

10:21

yes

John Rennie

$\nabla$ is a vector operator, also called the gradient:

Gradient

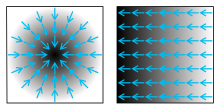
In vector calculus, the gradient is a multi-variable generalization of the derivative. Whereas the ordinary derivative of a function of a single variable is a scalar-valued function, the gradient of a function of several variables is a vector-valued function. Specifically, the gradient of a differentiable function f {\displaystyle f} of several variables, at a point P {\displaystyle P} , is the vector whose components are the partial derivatives of f {\displaystyle f}...

But it's far simpler than it looks because $\nabla V$ is just:

$$ \nabla V = \left( \frac{dV}{dx}, \frac{dV}{dy}, \frac{dV}{dz} \right) $$

i.e. it's a vector and the x, y and z components are just the derivatives of $V$ wrt x, y and z

Aladdin

Okay

John Rennie

$$ V = \frac{343}{r} = \frac{343}{\sqrt{x^2 + y^2 + z^2}} $$

Aladdin

That comes 7

49 sorry

John Rennie

So you just calculate the three derivatives, all of which are straightforward differentiation.

@Aladdin what is 49?

Aladdin

10:26

Lol I put values in there before diffrentiating

John Rennie

:-)

The three derivatives are all going to have the same form, so just calculate dV/dx then swap x for y and \z to get the remaining two.

$$ \frac{dV}{dx} = \frac{343x}{(x^2 + y^2 + z^2)^{3/2}} $$

Aladdin

It comes B

John Rennie

I haven't checked, but if it matches one of the answers that's a good sign :-)

Ah, 343 = 7^3

Aladdin

I think this one is more wholesome way for solving

John Rennie

That makes it easy :-)

@Aladdin I would do it this way as figuring out all the angles looks hard to me.

Aladdin

10:31

Yes

John Rennie

Have you come across operators like $\nabla$ before?

Nobody recognizeable

10:56

@JohnRennie hi.

John Rennie

@Nobodyrecognizeable hi

Nobody recognizeable

@JohnRennie how should I distinguish series and parallel dielectrics?

John Rennie

@Nobodyrecognizeable The two capacitors in the diagram have the same capacitance.

Transcript for