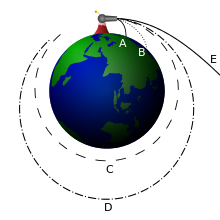
Newton's cannonball was a thought experiment Isaac Newton used to hypothesize that the force of gravity was universal, and it was the key force for planetary motion. It appeared in his book A Treatise of the System of the World.
== Thought experiment ==
In this experiment from his book (p. 5-8), Newton visualizes a cannon on top of a very high mountain. If there were no forces of gravitation or air resistance, the cannonball should follow a straight line away from Earth, in the direction that it was fired. If a gravitational force acts on the cannonball, it will follow a different path depending...