Mathematics - 2020-03-18 (page 1 of 2)

Ted Shifrin

00:07

@CaptainAmerica16 so what are we studying?

CaptainAmerica16

@TedShifrin Admittedly, I'm a little rusty. So I spent some time looking over my old Spivak problems and making sure I remembered how I got the answers. Today I just did a couple hours of logic stuff.

Oh, I also found an interesting problem in thomas calculus that I wanted to work on later

Don't ask for it because I already know you're not gonna think it's interesting XD

topologicalorientablesurface

is the Hausdorff dimension of the Sierpinski triangle, (side lengths 1), log3/log2?

@TedShifrin I meant up to one decimal place

What I did is the following: Observe that at any stage within the construction of the Sierpinski triangle, it is made up of 3 self similar copies, each of scale $\frac{1}{2}$. So, assuming $0<H^s(F)<\infty$ when $s=dim_HF$, we obtain $dim_HF=\frac{log2}{log3}$

Ted Shifrin

00:30

You just used a formula. You didn't explain it.

topologicalorientablesurface

@TedShifrin what do you mean? $H^s(F)$ is the hausdorff measure

Ted Shifrin

I know it's Hausdorff measure. We obtain how?

@CaptainAmerica: Maybe.

Oh, your two answers are upside-down from one another.

topologicalorientablesurface

@TedShifrin Right. $H^s(F)=H^s(F_1)+H^s(F_2)+H_s(F_3)$. Since each $F_i$ is similar to F with scale factor $\frac{1}{2}$, we get $H^s(F)=3\frac{1}{2}H^s(F)$. Assuming $0<H_s(F)<\infty$ when $s=dim_H(F)$, we get $dim_HF=\frac{log3}{log2}$

Leaky Nun

"log3/log2 up to one decimal place" that's a brand new phrase

topologicalorientablesurface

@Leaky I wasn't talking about $\frac{log3}{log2}$

lol

Leaky Nun

00:37

$H^s(F) = 3 (1/2)^s H^s(F)$, so $3 (1/2)^s = 1$, so $s = (\log(1/3))(\log(1/2)) = (\log 3)/(\log 2)$

you probably thought (log(1/3))/(log(1/2)) = (log 2)/(log 3) or something

topologicalorientablesurface

@LeakyNun right.

I fixed it.

Leaky Nun

so what's your question

topologicalorientablesurface

2 to be exact at this moment.. first.. is what I did correct? secondly, why can we make that assumption: "Assume $0<H_s(F)<\infty$ when $s=dim_HF"$

@LeakyNun

Ted Shifrin

The equation you typed above can only hold if $H^s=0$.

Leaky Nun

he forgot (1/2)^s

topologicalorientablesurface

00:43

yeah, I was thinking that something was off. I meant $H^s(F)=3\frac{1}{2^s}H^s(F)$

Leaky Nun

the parentheses are important.

Ted Shifrin

that, too.

CaptainAmerica16

@TedShifrin Suppose A and B are two neighborhoods of c. Prove that $A \cup B$ is a neighborhood of c.

Leaky Nun

draw a picture

topologicalorientablesurface

@CaptainAmerica16 union of open sets is open

Leaky Nun

00:45

there's a key step in the middle that requires a picture

CaptainAmerica16

yes, i know

topologicalorientablesurface

If $x\in A\cup B$ then $x\in A $ or $x\in B$. A,B are open. @CaptainAmerica16

CaptainAmerica16

@topologicalorientablesurface lol, I was just showing what I was gonna work on later.

topologicalorientablesurface

@CaptainAmerica16 oh, my bad. Sorry :P

For some reason I thought you were asking for help. My apologies @CaptainAmerica16

CaptainAmerica16

lol, it's cool

topologicalorientablesurface

00:58

@CaptainAmerica16 this might be of interest to you. In fact, you can also extend your proof to show that if $(A_i)_i$ is any collection (finite or otherwise) of neighborhoods of $c$ then $\bigcup_i A_i$ is also a neighborhood of c.

CaptainAmerica16

That actually does sound pretty interesting. I guess I could give it a shot

anakhro

@MikeMiller any cool topics in the intersection of geometry (manifolds and stuff) and functional analysis?

Ante

01:14

does someone knows why this simple sequence produces so much primes?

2

Q: Why is this sequence a good prime-generator?

For $n \in \mathbb N$ we can observe the $n$ remainders $b_1,...,b_n$ by writing $n$ as $n=a_k \cdot k+b_k$ for $1 \leq k \leq n$. Because of the familiar division-with-remainder theorem we have $0 \leq b_k <n$ Then the function $$r(n)=\sum_{k=1}^{\lfloor{\frac {n-1}{2}}\rfloor}b_k$$ can be def...

prime-numbers

Ted Shifrin

01:31

@anakhro: All sorts of index theory and PDE. None of which was ever my expertise.

Simple

do I just use the definition of product of measure

Leaky Nun

@Simple the question is certainly "how" you would use the definition

but you could prove a lemma that would help you

Simple

lemma?

Leaky Nun

01:49

yeah you heard that right, I said lemma

finding the right lemma is part of the exercise I guess

anakhro

@TedShifrin is index theory related to Morse theory in any way, or is it completely different?

topologicalorientablesurface

lol

you sure you were heard right? @LeakyNun

Leaky Nun

quite

@Ante answered

Ante

@LeakyNun i will read the answer now

i gave you +1 although i think some heuristics can be further explained

Ante

02:20

can $\sum_{k \mid n}$ be approximated with some closed-form-known-expression?

Leaky Nun

Divisor function

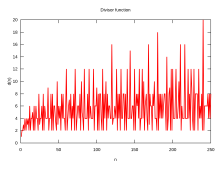
In mathematics, and specifically in number theory, a divisor function is an arithmetic function related to the divisors of an integer. When referred to as the divisor function, it counts the number of divisors of an integer (including 1 and the number itself). It appears in a number of remarkable identities, including relationships on the Riemann zeta function and the Eisenstein series of modular forms. Divisor functions were studied by Ramanujan, who gave a number of important congruences and identities; these are treated separately in the article Ramanujan's sum. A related function is the divisor...

it's a very important function in number theory

Ante

yes i know but i forgot that its approximation (loosely interpreted) is equivalent to RH

nbro

02:43

0

Q: What are the AI technologies currently used to help to solve the coronavirus pandemic?

The ongoing coronavirus pandemic of coronavirus disease 2019 (COVID-19), caused by severe acute respiratory syndrome coronavirus 2 (SARS-CoV-2), as of 17 March 2020, has affected more than 160 countries and territories (especially, in Europe), with more than 198,000 cases of COVID-19 have been re...

applications social healthcare real-world

uhoh

0

Q: Are there still no "simple analytical proofs" of Bloch's theorem in three dimensions?

Section 2.1.2 of Sydney G. Davison and Maria Stęślicka's 1992 Basic Theory of Surface States says: Bloch theorem The generalization of the Bloch-Floquet theorem to three dimensions is fairly obvious, and leads to the following form of the wave function $$\psi_\mathbf{k}(\mathbf{r}) ...

condensed-matter solid-state-physics electronic-band-theory

Math geek

02:58

A second family of characteristic curves comes from
$$\frac{dx}{x(c'_1y-2y^2)}=\frac{dy}{y(c'_1y-y^2-2x^3)}$$
The solution of this ODE is : $y=\frac{c'_1}{2}\pm \sqrt{x^3+\frac{(c'_1)^2}{4}+c_2}$

I tried to find the integration factor using the users.math.msu.edu/users/sen/Math_235/Lectures/lec_5s.pdf

Equation becoming complicated

Hopper

The question asks to find the rref of matrix A

I cant seem to figure this out. I know that if I put the given equation into a matrix I get [1 1 1 | 0] and I see there is 1 pivot, therefore by rank-nullity we know that the null space has to have dimension 2 (i.e. 2 independent vectors in the basis). But idk how to proceed or if Im doing this correctly.

user76284

03:41

Are collection, union, powerset, and infinity sufficient to interpret ZFC?

Leaky Nun

04:30

what is "interpret"?

Secret

04:55

I have been thinking about some nondual logic

I have $X \implies Y$ where $\implies$ is to be understood intuitively as "leads to" as in it is an antisymmetric relation

and I also have $\text{all non} X \implies Y$

where "non" includes all possible strings made of "not"

that is anything but $X$

I am trying to find a formula $\phi$ such that $\phi(X) \implies Y$ is non true

that is $\phi(X)$ would fall outside the two givens and hence cannot be said to evaluate to $Y$ by "leads to"

Ante

i have been thinking about assigning to implication two different truth tables, but still cannot find an example to justify that, if one exists at all

Guru Vishnu

09:19

> Suppose there are $r$ things to be arranged, allowing repetitions. Let further $p_1,p_2,\dots p_r$ be the integers such that the first object occurs exactly $p_1$ times, the second occurs exactly $p_2$ times, etc. Then the total number of permutations of these $r$ objects to the above condition is $$\frac{(p_1+p_2+\dots+p_r)!}{p_1!p_2!\dots p_r!}$$

It seems $p_1+p_2+\dots+p_r\neq r$. So, I think the above formula doesn't count cases in which only one object of one kind is present and nothing else. Could anyone clarify whether I understood this properly? I understood the formula $\frac{n!}{p!q!}$ to determine the number of ways to arrange $n$ objects which contains two identical types of counts $p$ and $q$ each, where $p+q=n$

Or if possible, could anyone explain what the above formula means?

Thank you.

Ante

@LeakyNun

https://math.stackexchange.com/questions/3585239/awesome-number-13-phenomenon

Semiclassical

13:37

@GuruVishnu for googling purposes, that ratio is what's known as a multinomial coefficient, by analogy with the binomial coefficient which you also reference

as a starting point, consider the case of $r=3$ and note the following:

$$\frac{(p_1+p_2+p_3)!}{p_1!p_2!p_3!}=\frac{(p_1+p_2+p_3)!}{p_1!(p_2+p_3)!}\cdot \frac{(p_2+p_3)!}{p_2!p_3!}$$

that is, you can interpret the left-hand term in the following way: "the number of ways to choose $p_1$ out of $p_1+p_2+p_3$ objects" times "the number of ways to choose $p_2$ out of $p_2+p_3$ objects"

which, if you think it it through, is exactly how you'd count the number of ways to take $p_1+p_2+p_3$ objects and divide them into boxes containing $p_1,p_2,p_3$ objects respectively

so that justifies the case of $r=3$. it's not too hard to turn that into a proof by induction if one puts in the work

Abhas Kumar Sinha

14:06

@Semiclassical Good Evening sir.

Semiclassical

hi

Abhas Kumar Sinha

@Semiclassical sir, : swarajyamag.com/ideas/…

Is the math in the second point there, weak law of large numbers ?

or what?

@Semiclassical Sampling thing...

Semiclassical

could not tell you---I'm not someone with much intuition for practical statistics, particularly as far as biostatistics goes

Abhas Kumar Sinha

@Semiclassical no that's related to datascience, ML, not biology...

Semiclassical

biostatistics covers medical sampling as well

Abhas Kumar Sinha

14:13

That was general sampling I guess.... :O

skillpatrol

this far into the pandemic and this room has no activity :(

Bioinformatics

General discussion for bioinformatics.stackexchange.com

Abhas Kumar Sinha

so sad <(")

Semiclassical

14:41

@Knight i'm here now, but I'm not sure I have the brainpower to follow Griffiths' derivation for a toroid's magnetic field right now

i remember it being rather involved

Knight

@Semiclassical I was just about to ask you

@Semiclassical No problem sir, I will ask it when you will be in a good mood :-)

Semiclassical

my recollection is that, to avoid headaches, one assumes that the observation point is at $\vec{r}=(x,0,z)$ with $x>0$

Knight

Yes that’s the point where we want our field

Semiclassical

if you can figure it for a point in that region, then you can figure it out everywhere else via rotational symmetry

Knight

Yes

Abhas Kumar Sinha

14:45

calculus

Semiclassical

@knight take a look at the conventions on the back cover for (I think? maybe front cover) for the basis elements in spherical/cylindrical coordinates

I believe you have $\hat{s}=\cos\phi~\hat{x}+\sin\phi ~\hat{y}=\langle \cos\phi,\sin\phi,0\rangle$

Abhas Kumar Sinha

@Semiclassical laplace in spherical coordinates?

Semiclassical

no. just how to write vectors like $\hat{s}$ in terms of Cartesian coordinates

Knight

Yes

Abhas Kumar Sinha

k

Knight

14:49

I understand that

Sir May I ease your work, if you allow?

Semiclassical

ok?

Abhas Kumar Sinha

use Aragand plane and just write, $ae^{ i\theta}$ that's easy while computation.

Semiclassical

the point is that you're looking at the current vector $\vec{I}$ at the source point $\vec{r}'$

if $\vec{r}'$ were on the positive $x$-axis, then the current vector would be $I\hat{y}$

Knight

Semiclassical

if $\vec{r}'$ were on the positive $y$-axis, then instead the current vector is $I\vec{y}$

ah. hmmm

Knight

14:52

Is my blue arrow correctly depicting the current vector?

How he got the angle that current vector will make with $x-$ axis?

Semiclassical

oh, i see it now. yes, that looks right. $\vec{I}$ has a vertical component and a radial component

imagine looking at that scenario from the top

then your blue current vector would point towards the center of the toroid

Knight

Okay

@Semiclassical Very hard to imagine that

Yeah yeah yeah! I got you, when we look from top the only component we would see is the radial component ,ha?

Semiclassical

right

and if you look at $\vec{r}'$ from that same point of view, then it also points along that same direction (but outwards rather than inwards)

and, by assumption, $\vec{r}'=\langle x',y',z'\rangle = s'\hat{s}+z'\hat{z}$

in particular, $\vec{r}'$ has no $\phi$ component

and neither does $\vec{I}$. (if it did, then from the top down they wouldn't point in the radial direction)

that means that the only thing we can really say about the current vector is that it's of the form $I_s \hat{s}+I_z\hat{z}$

no $\hat{\phi}$ component

and since $\hat{s}$ is already a function of $\phi$, then so too must $\vec{I}$.

Knight

@Semiclassical Sir $\vec{r'}$ does have a $\phi$ component

it goes up, radially outwards and then rotates about the x axis

Semiclassical

no. it goes up, radially outwards, then down, then radially inwards

well, i say that

as a matter of engineering, a toroid looks like this:

which, you'll note, is not strictly "up, radially outwards, down, and then radially inwards to form a loop"

Knight

15:11

Yes, it goes a little in the $\phi$ direction as we move from one loop to another

Semiclassical

that said, for the purposes of Griffiths' derivation, he is very much neglecting that small $\phi$ component

the idea being that, while there is a small $\phi$ component, it's sufficiently small for these purposes that it can be ignored

for engineering purposes, of course, this may not be at all satisfactory

Knight

He is considering that the coil or the loop are essentially separate and closed

Semiclassical

right. another way to say it is that he's treating the current as a surface current on the surface of the toroid

specifically, one which has no circumferential componnent

Knight

Yes

Semiclassical

that said, the situation is similar with a solenoid

Knight

15:15

yes

Semiclassical

in the solenoid case, you ignore any longitudinal current

Knight

Yes

Semiclassical

if you now imagine bending a solenoid around to make a toroid, then that goes to having no circumferential current

real solenoids and real toroids are not so simple, of course, but Griffiths is a physics book not an engineering text :P

Knight

:)

Yeah, we turned the solenoid horizontally and then we connected its ends, ha?

Semiclassical

yeah (and contorted it quite a bit)

Knight

15:18

:D

Semiclassical

another way to say it: suppose you made a toroid with $a\approx b\gg 0$

so it's a very large 'loop' but a small circular cross-section

in that case, if you pick any portion of the toroid, then it will look approximately straight

and therefore will approximate a solenoid

so in that sense you could view a solenoid as a special case of a toroid

Knight

Yes, but is that approximation necessary for the present case?

Semiclassical

yes. otherwise, he wouldn't be able to say "forget about the phi component"

Whether that's a -good- approximation of reality is another matter enntirely. i don't rightly know

Knight

All right!

Semiclassical

but it's certainly a common approximation and (as the solenoid comparison shows) not that strange of one

one could probably do the computation regardless, but i've never tried and I don't plan on doing so now :P

Knight

15:23

:D

Semiclassical

actually, i don't think it even matters:

Knight

But still $\vec{r'}$ have a $\phi$ component, I think

Semiclassical

...hmm. hmmmmmmm

no, i'm not convinced

it should matter, because if you had a toroid whose surface current is purely circumferential

then surely the magnetic field should be nonzero

"surely"

Knight

Yes, but the position of current may have a $\phi$ component

Semiclassical

well, that's what I'm saying: suppose you consider the opposite idealization, where the current is assumed to only have a $\phi$ component

it seems like this would still generate a magnetic field

Knight

15:27

I'm unable to imagine how would a figure look with only $\phi$ component

Semiclassical

basically, consider your current as a bunch of horizontal loops around the z-axis

arranged so that the loops form a toroid

Knight

Finding it hard to think of

Semiclassical

My suspicion, anyways, is that this still doesn’t matter so long as you look inside the toroid

I’m not 100% on that

Knight

okay

Semiclassical

a remarkable amount of hedging, I know

That said: this is in the context of finding B via the Biot-Savart law, right?

Knight

15:32

Yeah, sometimes simplicity makes things complex

@Semiclassical YEAH

Semiclassical

You’ll eventually see the derivation in terms of Ampere’s law

Knight

Yes

Semiclassical

And I think in the case the irrelevance of $I_\phi$ is a lot more apparent

As a comparison:

Knight

We're using Biot-Savart law is used only to get the direction $\mathbf B$

Semiclassical

If I have a uniformly charged thin spherical shell, then I can compute the electric field inside in two ways

One is to use Coulomb’s law and compute a complicated double integral over the sphere

The other is to use Gauss’s law and symmetry, with which you immediately conclude that the there’s no field inside

Knight

15:35

Or use the Gauss' law

Semiclassical

Right

In both cases, you get the same answer: E=0 inside

But in the first method that may seem like a miracle, whereas in the second case it’s obvious

So the analogy here would be: it may be that the phi component of the current is irrelevant to the field inside a toroid, but that this is much harder to see via Biot-Savart vs Ampere

Knight

yes

Semiclassical

Id have to look at his derivation of a toroid vs Ampere, tho

Knight

You mean Andre Marie Ampere himself did the derivation ?

Semiclassical

No. I mean: I’d have to see again how Griffiths handles the derivation of a toroid’s magnetic field using Ampere’s Law

Knight

15:43

Okay. You need the images or you got the copy?

Semiclassical

I’d need to dig out my copy of Griffiths.

But in the meantime I’d suggest looking that up in yours, to see what if anything he says about it

Knight

Semiclassical

Hmm. I see your point

Brb

Knight

This thread may help you in helping me :D

Semiclassical

To ensure that he can use Ampere’s law in a simple way, he derives that the B-field inside a toroid is circumferential. But that derivation assumes that the current has no circumferential component

Knight

15:56

Yes

Balarka Sen

Margulis got an Abel prize

Semiclassical

So it’s not at all clear whether it still applies if you account for the winding angle

Knight

It's too controversial :D

Semiclassical

My guess is that it does, at least inside a toroid. But I’m basing that on an analogy with the infinite solenoid which may not be valid

Knight

Well, it's a rule of symmetry: Circumferential current will produce a circumferential field, one-directional current will produce one-directional field

Semiclassical

16:03

I think the safest statement is that he assumes that the phi-component may be neglected

Knight

YES

Semiclassical

Oh, I see where he assumes it

@Knight take a look at the second sentence of his problem statement

Knight

Yes

59 mins ago, by Knight

He is considering that the coil or the loop are essentially separate and closed

Semiclassical

“The winding us uniform and tight enough that each turn may be considered a closed loop.”

Right. So that was built into the problem from the start

Knight

Yes

Semiclassical

16:12

I hadn’t seen that and thought he was just being sloppy :P

Knight

Every loop is separate and hence is closed

Semiclassical

right

huzzah for simplifying assumptions

Knight

huzzah! huzzah! I like pronouncing this word

Semiclassical

lol

Knight

huzzah

topologicalorientablesurface

16:16

is every Borel unbounded set the countable union of bounded sets?

Knight

@Semiclassical Sir I have to go, may I?

Semiclassical

of course

i'm not holding you here any more than you're holding me here :P

Knight

Thank you

topologicalorientablesurface

For hausdorff measure, is it always true that $H^s(F)\geq s?$

Alessandro Codenotti

What's $F$? The $s$ in $H^s$ means the $s$-dimensional measure?

Thorgott

16:28

$B=\bigcup_{n=1}^{\infty}(B\cap(-n,n))$

every unbounded set is a countable union of bounded sets

depending on whether you mean bounded in the sense of measure or bounded in the sense of metric, the same idea generalizes to $\sigma$-finite or $\sigma$-compact spaces respectively

topologicalorientablesurface

@Thorgott in general metric spaces?

whats the hausdorff dimension of $dim_H\mathbb{R}^n$

Im feel like its n

but unsure how to prove it

Thorgott

16:44

that's equivalent to asking whether every metric space is the countable union of bounded subsets, which I would suspect is false, but I actually don't know

also yes, the Hausdorff dimension of $\mathbb{R}^n$ is $n$

Alessandro Codenotti

@Thorgott Replace $(-n,n)$ with the ball of radius $n$ around an arbitrary $x_0$ in the metric space

You might need uncountably many bounded sets if you require them to be uniformly bounded though

Thorgott

oh, I shouldn't have missed that..

Alessandro Codenotti

Hi @Ted

Archer

17:06

Any course recommendations for Complex Analysis (Introductory) ?

The MIT course is black and white and the video quality is bad :/

Simple

17:30

Prove that there exists a set $A\subset\mathbb{R}$ such that $m^{*}(G\setminus\,A)=\infty$ for every open set $G$ contains $A$

Alessandro Codenotti

Like $\Bbb Q$?

Wait is that an outer measure?

Simple

$\mathbb{Q}$ has measure zero,

Alessandro Codenotti

Hi @Lukas

@Simple I agree

($\Bbb Q$ doesn't work, no countable set can work)

Actually no Lebesgue measurable set of finite measure can work

topologicalorientablesurface

How do I should that the hausdorff measure of an n dimensional ball is at least n?

if $s>n$ then $H^s(B(x,r))=0$ and so $dim_HB(x,r)\leq s$

Alessandro Codenotti

On $\Bbb R^n$, $H^n$ agrees with the Lebesgue measure and a ball has positive lebesgue measure

topologicalorientablesurface

17:40

I don't see how to proceed @AlessandroCodenotti

we briefly discussed lebesque measures

Alessandro Codenotti

What don't you see exactly?

topologicalorientablesurface

why $dim_HB(x,r)\geq n$

Alessandro Codenotti

Because $H^n(B)>0$

topologicalorientablesurface

aha, so it has finite "volume"

n dimensional volume, I mean

we were told to think about this intuitively

Alessandro Codenotti

Intuitively if $\Bbb R^n$ didn't have dimension $n$ for any notion of dimension we wouldn't be calling it a dimension haha

topologicalorientablesurface

17:48

I agree. But I keep trying to formalize my intuition, hence the questions.

feynhat

Hello people.

Have your schools/colleges been shutdown?

Alessandro Codenotti

(this applies to the Hausdorff dimension, Lebesgue covering dimension, small and large inductive dimension, asymptotic dimension etc.)

topologicalorientablesurface

@feynhat no. Everythings online though.

Alessandro Codenotti

@feynhat My country has been shutdown!

topologicalorientablesurface

@AlessandroCodenotti thanks. In fractal geometry, we're only focusing with minkowski and hausdorff

feynhat

17:50

@topologicalorientablesurface US? @AlessandroCodenotti Italy?

topologicalorientablesurface

@AlessandroCodenotti How should I think about the lebesque measure? Just as a generalization of volume, length and area?

Alessandro Codenotti

@feynhat Yes

feynhat

@AlessandroCodenotti Stay safe man...

Alessandro Codenotti

@topologicalorientablesurface Yes. You start with some intuitive properties, namely $m((a,b))=b-a$, translation invariance etc. and see to how many set you can assign a measure without running into issues

Simple

If $A$ is a non-lebesgue measure set, $m^{*}(G\setminus\,A)>...$, I am not sure

topologicalorientablesurface

17:54

@AlessandroCodenotti can a non-empty set $F$ in $\mathbb{R}^n$ ever have 0 lebesque measure?

Alessandro Codenotti

@topologicalorientablesurface Any countable set

And many uncountable ones, planes in $\Bbb R^3$, or anything "lower dimensional" like that

topologicalorientablesurface

@AlessandroCodenotti can a non-empty uncountable set $F$ in $\mathbb{R}^n$ ever have 0 lebesque measure>

oh right.

Alessandro Codenotti

@topologicalorientablesurface Yes, even in $\Bbb R$, the Cantor set has measure $0$

topologicalorientablesurface

Hmm. What makes the ball have positive lebesque measure?

@AlessandroCodenotti

Alessandro Codenotti

Intuitively it should have some volume since it is full

topologicalorientablesurface

17:57

@Alessando right. Does the lebesque measure coincide with the diameter?

of the ball?

feynhat

@topologicalorientablesurface No, not in general. But it does for n=1.

Alessandro Codenotti

@topologicalorientablesurface With the volume

topologicalorientablesurface

@feynhat Right. So I should think of anything with the same "dimension as n" in $\mathbb{R}^n$ as having positive volume, anything less than that 0, anything more, infinity?

@AlessandroCodenotti

well that would mean that the hausdorff dimension of a ball in $\mathbb{R}^n$ is equal to n, right?

Alessandro Codenotti

@topologicalorientablesurface yes

feynhat

What is the Hausdorff dimension of Cantor set?

topologicalorientablesurface

18:03

@feynhat $\frac{log2}{log3}$

oops

no, thats right

feynhat

I see.

topologicalorientablesurface

It has two copies of itself, of scale $\frac{1}{3}$. Assuming $0<H^s(F)<\infty$ when $s=dim_HF$, we obtain $dim_H(Cantor set)=\frac{log2}{log3}$

@feynhat

So, any open set in $\mathbb{R}^n$ has $n$ dimensional hausdorff measure.

I haven't taken measure theory yet. But it does seem interesting.I suppose ill look more into it after im done with classes

feynhat

@AlessandroCodenotti Hey, can you help me with the proof of homotopy lifting criterion?

$p : (\tilde X, \tilde x_0) \to (X, x_0)$ is a covering map, and $Y$ is connected, locally-path connected. We want to construct a lift $\tilde f$ of $f : (Y, y_0) \to (X, x_0)$.

Alessandro Codenotti

It's been waaay too long since I saw those things to be of help I'm afraid

feynhat

We define $\tilde f(y)$ as follows: suppose $\gamma$ is a path joining $y_0$ to $y$. We push this path to $X$, then lift it to $\tilde X$ and choose the endpoint of the lifted path, that is $\tilde f (y) = \widetilde {f \circ \gamma} (1)$.

I am trying to understand why this is well-defined.

Suppose we choose some other path $\gamma'$. We want to show $\widetilde{f\circ\gamma}(1) = \widetilde{f\circ\gamma'}(1)$.

@AlessandroCodenotti I will try and state my problem (and solution) in terms of just paths and homotopies... No point-set topological nonsense.

Forget about the lifting for a moment.

Alessandro Codenotti

18:25

It's dinner time here, I have to leave for a while, sorry

feynhat

Ahh... okay. No worries.

topologicalorientablesurface

@feynhat why don't you like point set topology? :(

feynhat

@topologicalorientablesurface I like point-set topology. By 'nonsense' I meant that there are a lot of abstract definitions which are sometimes not so natural that you can recall out of the top of your head.

Balarka Sen

18:48

@feynhat You need a condition there, $f_* \pi_1(Y, y_0)$ goes inside the subgroup $p_* \pi_1(\widetilde{X}, x_0)$ of $\pi_1(X, x_0)$. Otherwise in general there is no such lift.

Indeed, your thing is well defined precisely because of this condition.

feynhat

@BalarkaSen Yeah. I forgot about that one.

Balarka Sen

Tell me why this resolves your problem

feynhat

Consider the loop at $x_0$ which traverses $\widetilde{f\circ\gamma}$ followed by $\widetilde{f\circ\gamma'}$ in reverse, the class of this loop is in $p_*\pi_1(\widetilde X, \widetilde x_0)$, by your condition.

Balarka Sen

Truly.

feynhat

The lift of every loop whose class belong to that subgroup is again a loop, right?

By 'that subgroup', I mean $p_*\pi_1(\widetilde X)$

Bioinformatics

Transcript for

$Mathematics$ Mathematics