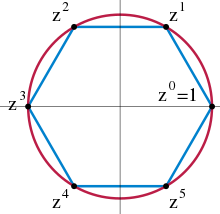
In group theory, the direct product is an operation that takes two groups G and H and constructs a new group, usually denoted G × H. This operation is the group-theoretic analogue of the Cartesian product of sets and is one of several important notions of direct product in mathematics. In the context of abelian groups, the direct product is sometimes referred to as the direct sum, and is denoted G ⊕ H. Direct sums play an important role in the classification of abelian groups: according to the fundamental theorem of finite abelian groups, every finite abelian group can be expressed as the direct...