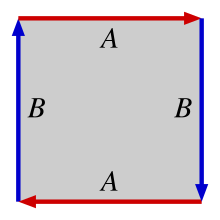
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215
user84215