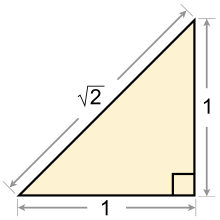
In geometry and algebra, a real number r is constructible if and only if, given a line segment of unit length, a line segment of length |r| can be constructed with compass and straightedge in a finite number of steps. Not all real numbers are constructible and to describe those that are, algebraic techniques are usually employed. However, in order to employ those techniques, it is useful to first associate points with constructible numbers.
A point in the Euclidean plane is a constructible point if it is either endpoint of the given unit segment, or the point of intersection of two lines determined...