0
Let $A$ be a collection of subsets of $\{1,2,\dots,n\}$ that is closed under taking subsets (that is, if $U\in A$ and $V\subseteq U$ then $U\in A$). Is there always an involution $f:A\to A$ such that $f(V)\cap V=\emptyset$ for all $V\in A$? I'm guessing yes.
Note that if $A=\mathcal P(\{1,2,\dot...
I sometimes have to endure my parents' or teachers' endless scolding, and sometimes endure endless lectures on boring things in school, and occasionally endure really long trips.
One way for me to deal with them is to do mental arithmetic, such as calculating squares and cubes, and approximatin...
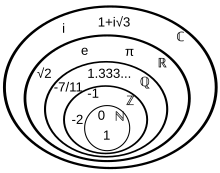
A number is a mathematical object used to count, measure, and label. The original examples are the natural numbers 1, 2, 3, 4 and so forth. A notational symbol that represents a number is called a numeral. In addition to their use in counting and measuring, numerals are often used for labels (as with telephone numbers), for ordering (as with serial numbers), and for codes (as with ISBNs). In common usage, number may refer to a symbol, a word, or a mathematical abstraction.
In mathematics, the notion of number has been extended over the centuries to include 0, negative numbers, rational numbers...
user84215
user84215
In mathematics, a projective plane is a geometric structure that extends the concept of a plane. In the ordinary Euclidean plane, two lines typically intersect in a single point, but there are some pairs of lines (namely, parallel lines) that do not intersect. A projective plane can be thought of as an ordinary plane equipped with additional "points at infinity" where parallel lines intersect. Thus any two distinct lines in a projective plane intersect in one and only one point.
Renaissance artists, in developing the techniques of drawing in perspective, laid the groundwork for this mathematical...
user84215
In geometry, an affine plane is a system of points and lines that satisfy the following axioms:
Any two distinct points lie on a unique line.
Each line has at least two points.
Given any line and any point not on that line there is a unique line which contains the point and does not meet the given line. (Playfair's axiom)
There exist three non-collinear points (points not on a single line).
In an affine plane, two lines are called parallel if they are equal or disjoint. Using this definition, Playfair's axiom above can be replaced by:
Given a point and a line, there is a unique line which contains...
user84215
