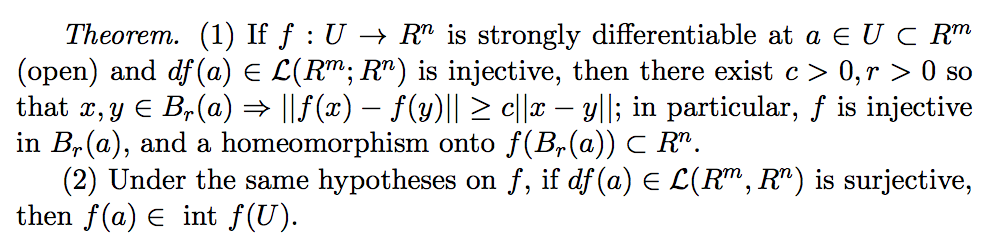user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700
user228700