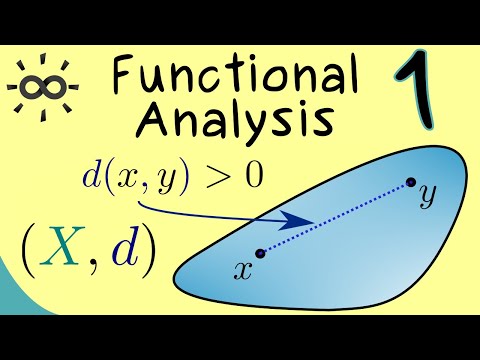
Modern Abstract Analysis
For functional analysis, measure theory, and related areas. MathJax in chat: http://www.math.ucla.edu/~robjohn/math/mathjax.html
256d ago – Martin Sleziak
26
export all events for this room