15 messages found
Thanks for posting here before unilaterally creating a new tag. I'm unfamiliar with the subject area and don't have a strong opinion one way or the other about its creation. Here are some topics that often come up when doing tag management (see this Meta Math SE post for examples).
What is the ...0
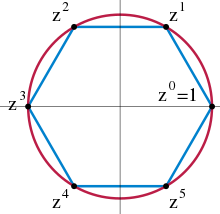
In mathematics, a Kleinian group is a discrete subgroup of the group of orientation-preserving isometries of hyperbolic 3-space H3. The latter, identifiable with PSL(2, C), is the quotient group of the 2 by 2 complex matrices of determinant 1 by their center, which consists of the identity matrix and its product by −1. PSL(2, C) has a natural representation as orientation-preserving conformal transformations of the Riemann sphere, and as orientation-preserving conformal transformations of the open unit ball B3 in R3. The group of Möbius transformations is also related as the non-orientation...
In mathematics, a convergence group or a discrete convergence group is a group
Γ
{\displaystyle \Gamma }
acting by homeomorphisms on a compact metrizable space
M
{\displaystyle M}
in a way that generalizes the properties of the action of Kleinian group by Möbius transformations on the ideal boundary
S
2
{\displaystyle \mathbb {S} ^{2}}
of the hyperbolic 3-space
...
Definition: For a subgroup $G$ of the group $PSL(2,\mathbb{C})$ acting on $\mathbb{P}^1$, its domain of discontinuity is the set of all points, $z$, with the following properties:
$1.$ The stabilizer $G_z$ of $z$ is finite.
$2.$ $\exists U$, a neighbourhood of $z$ such that,
$\space \space\...kleinian